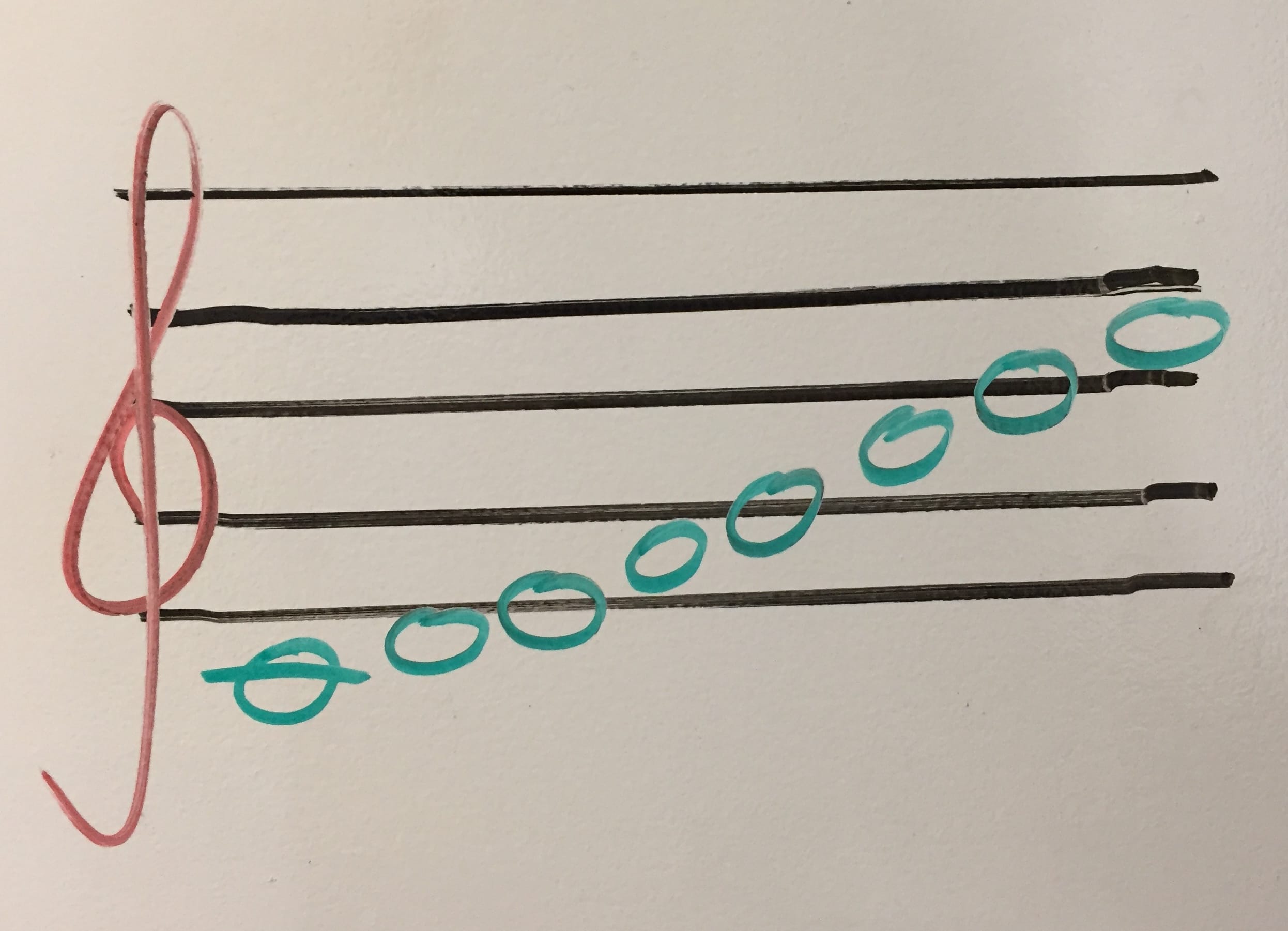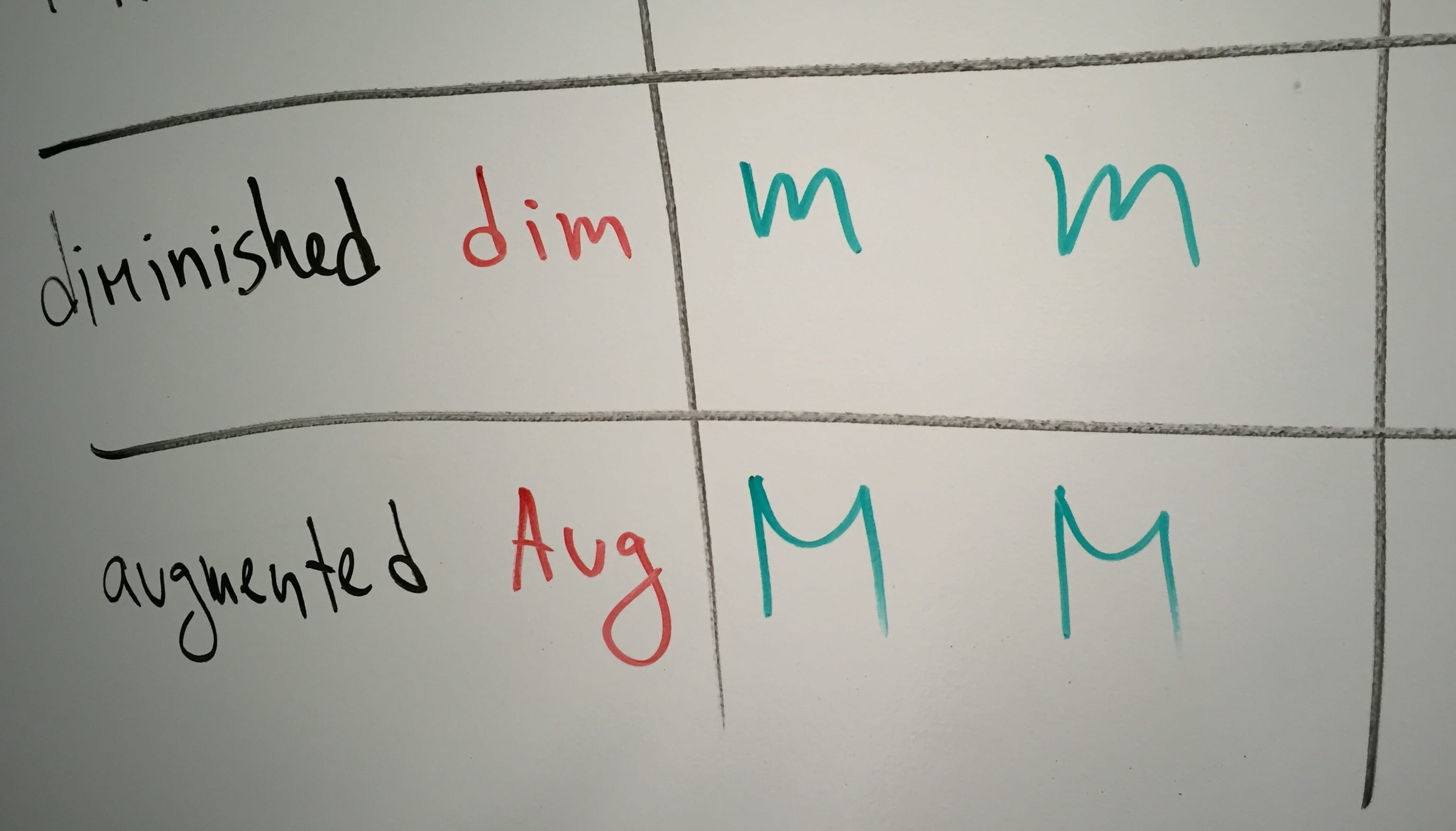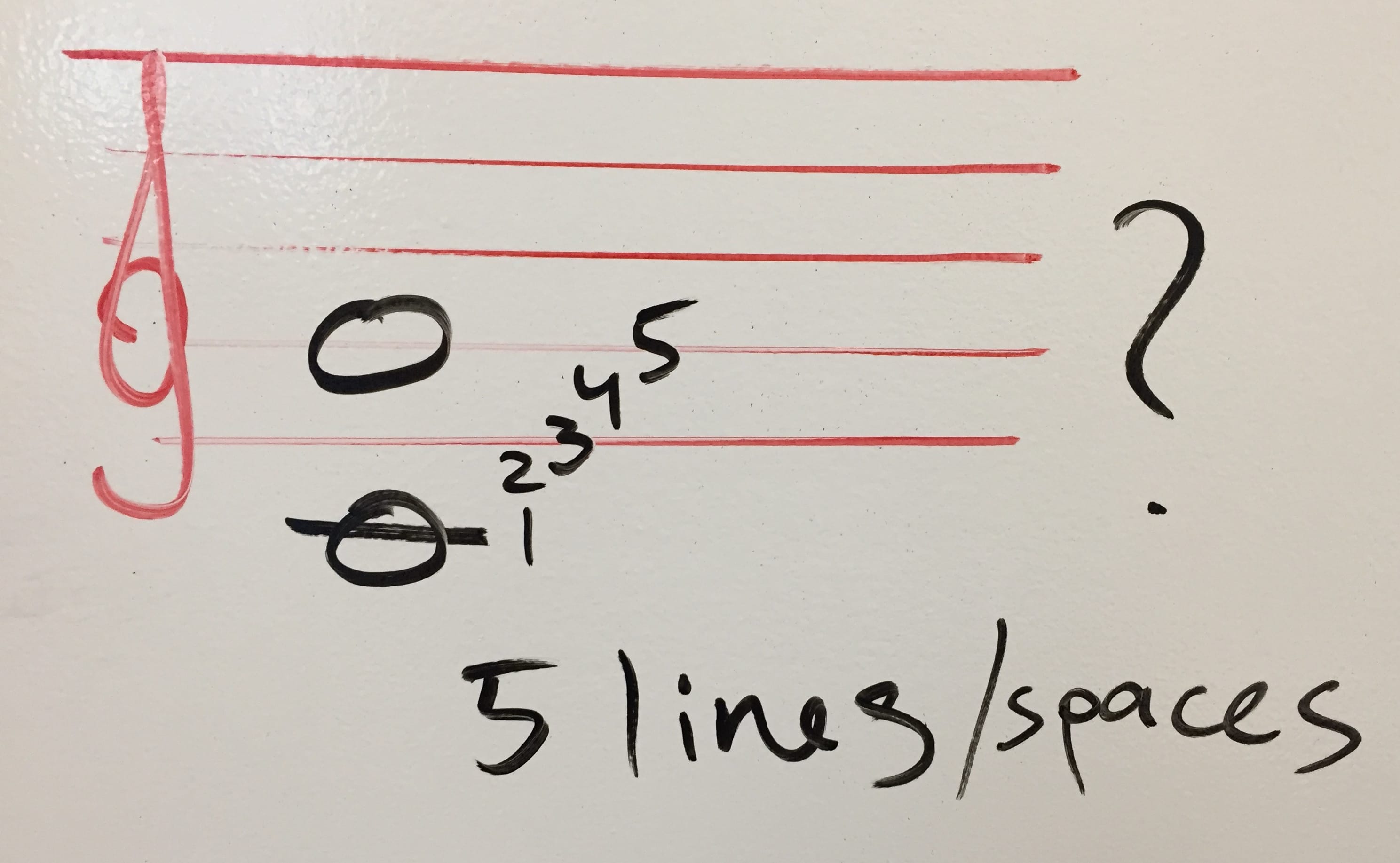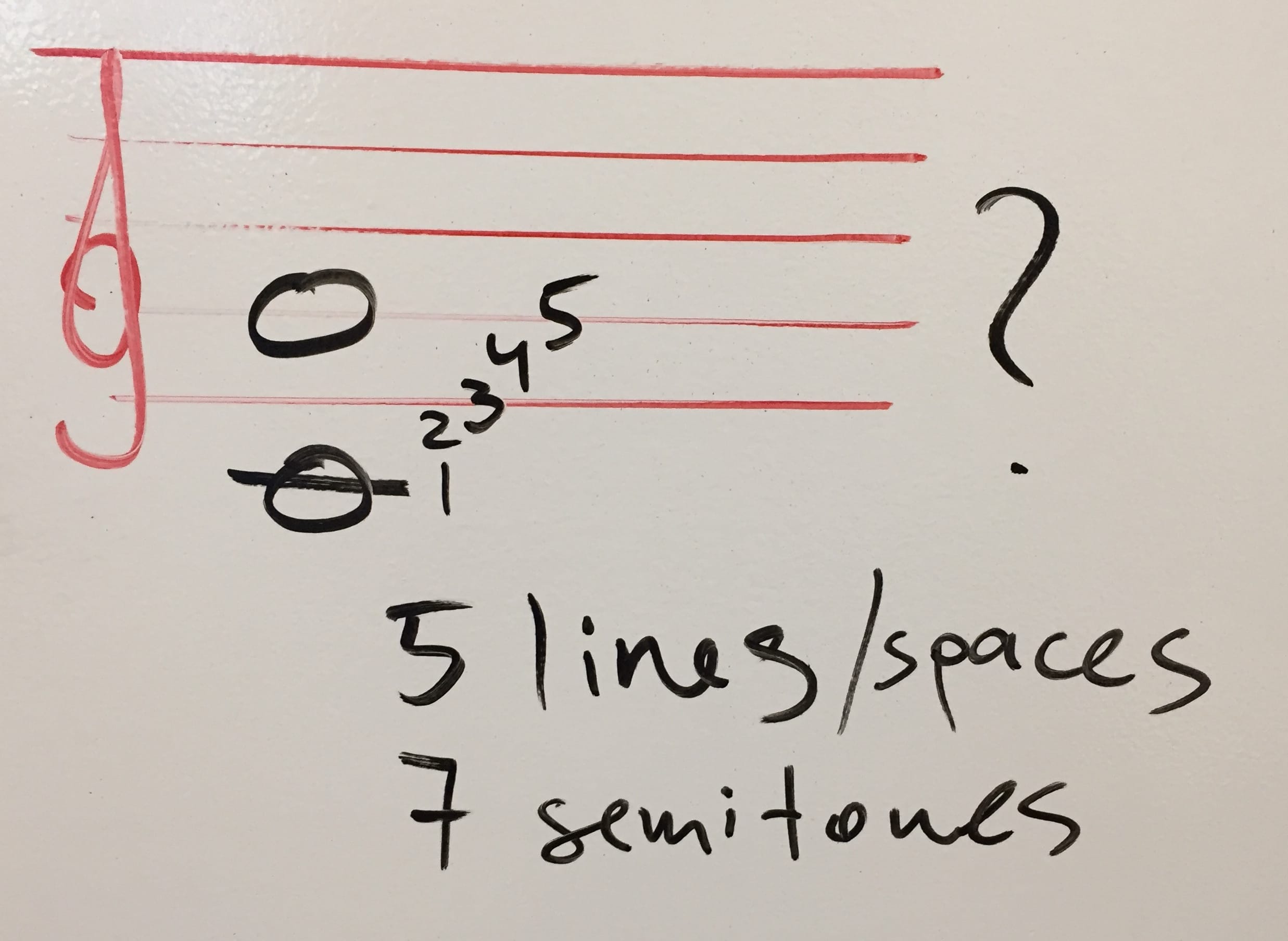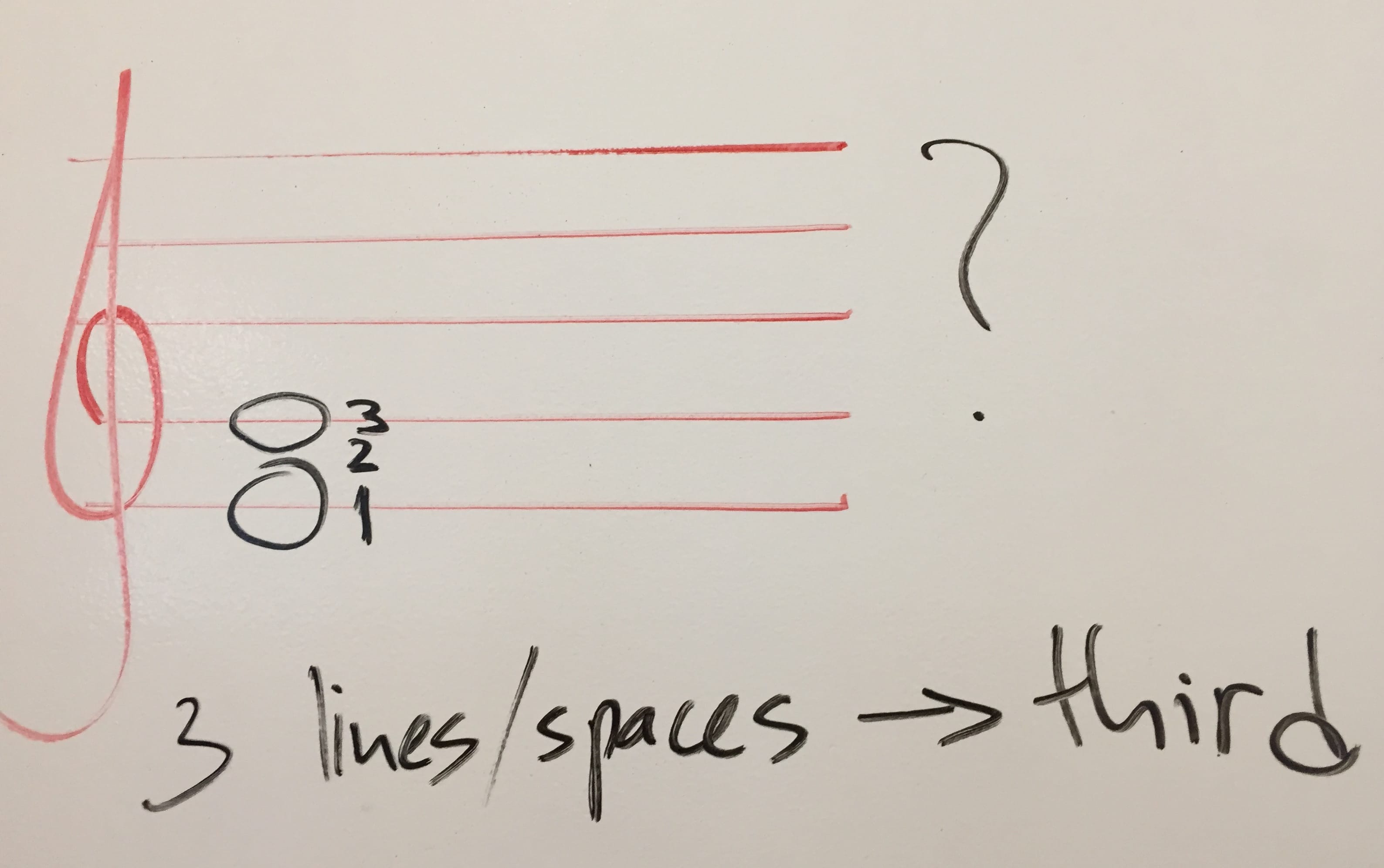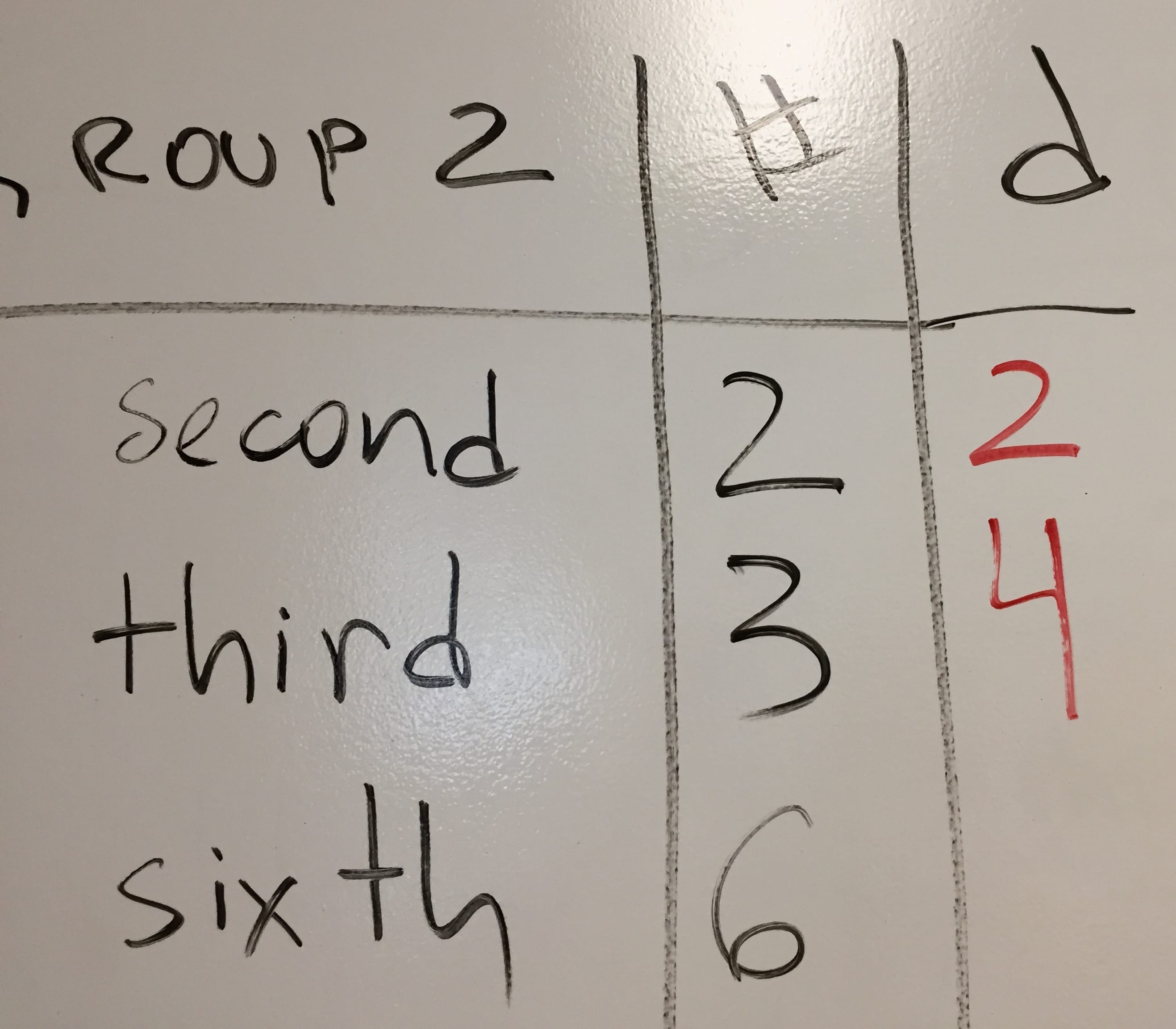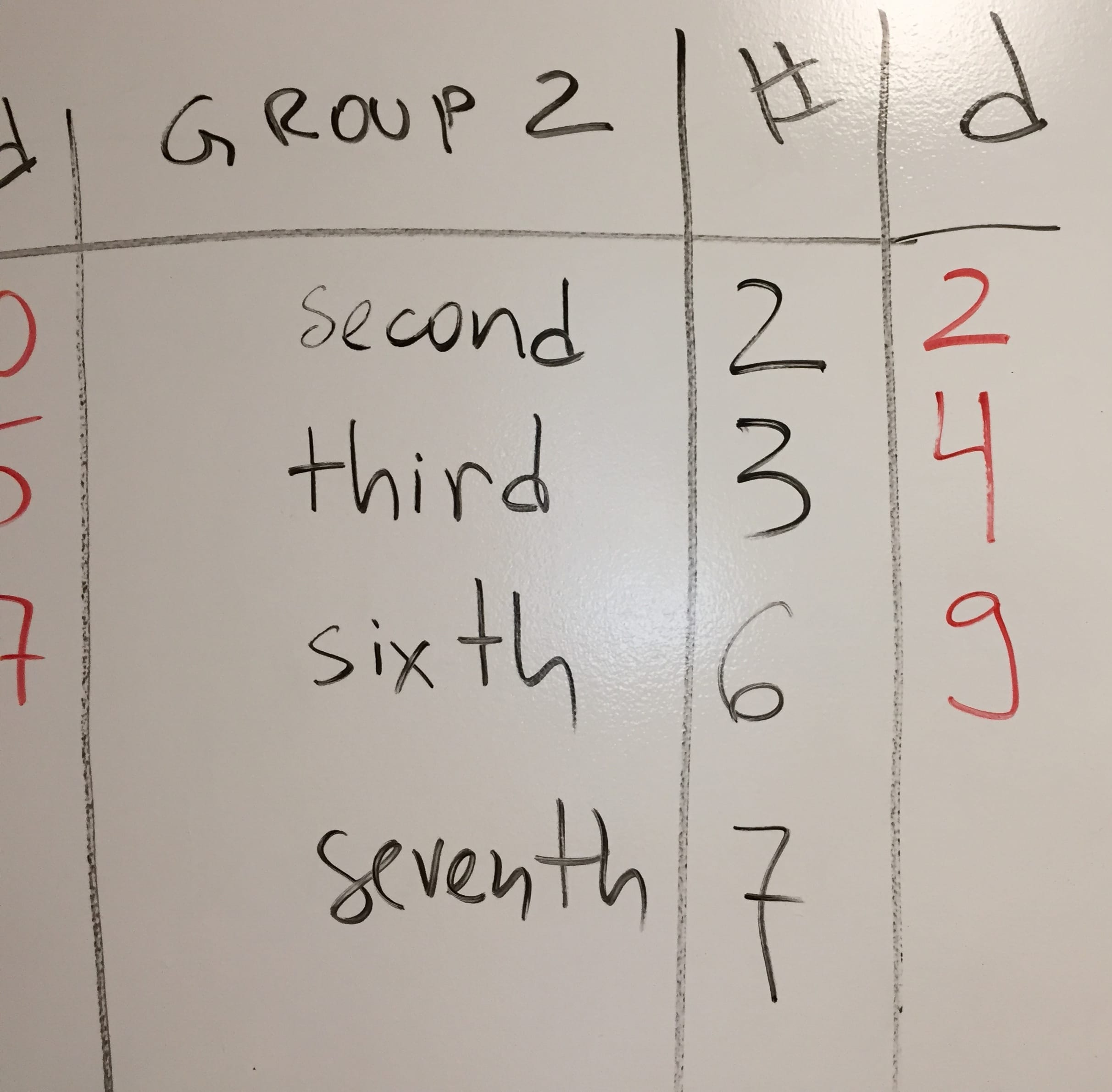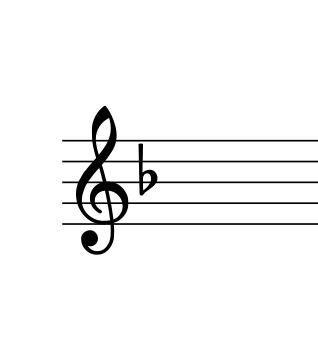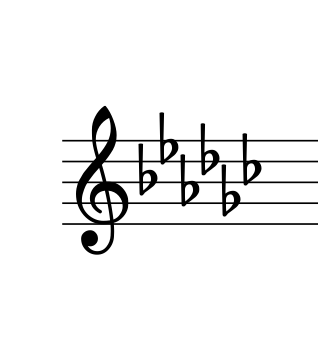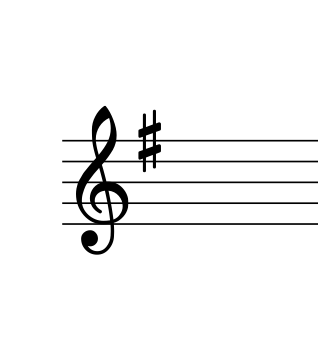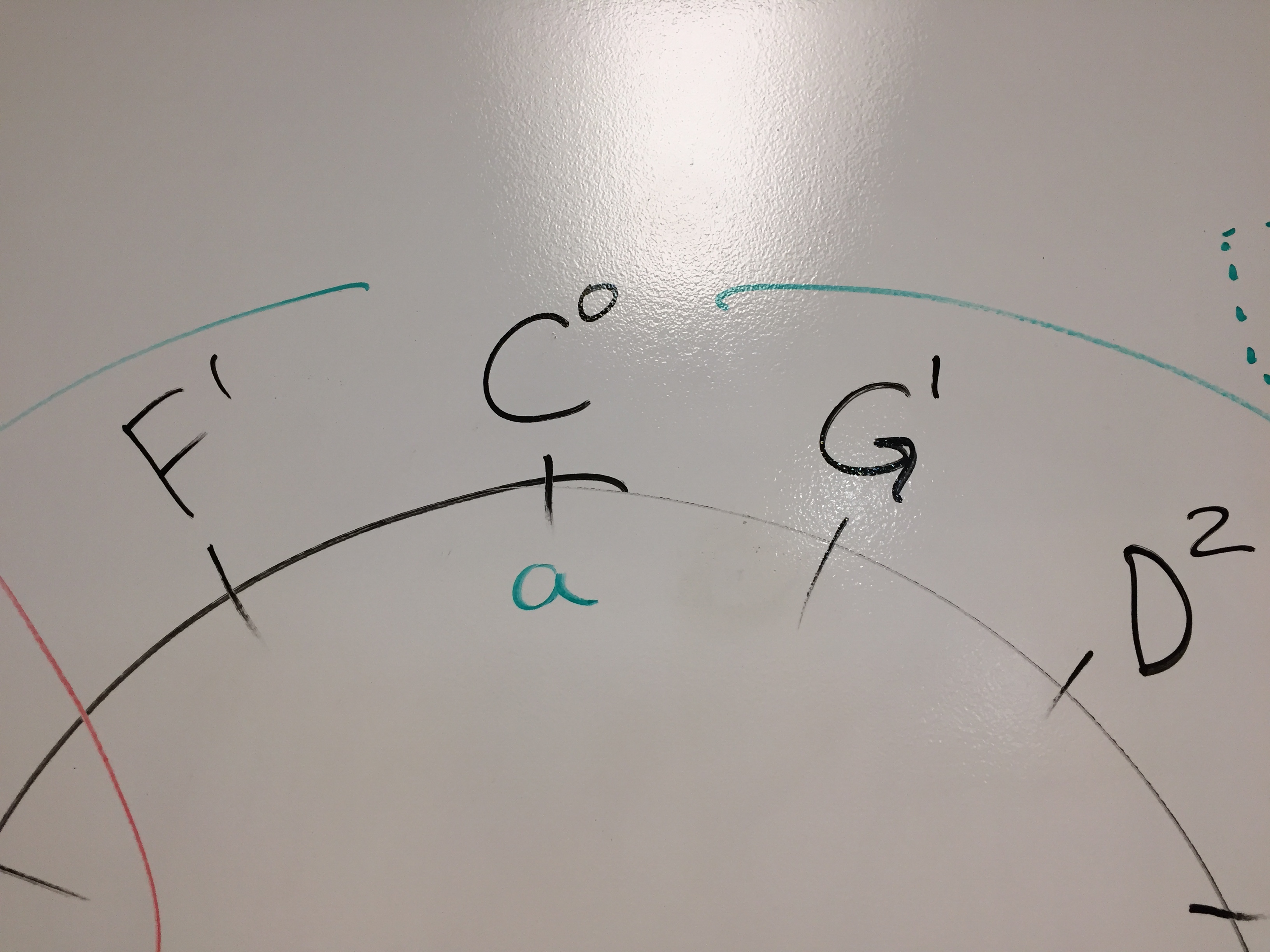Author: admin

I’m taking Music courses in the local Santa Monica College. It’s awesome! The teachers are really dedicated and into it, the students are so talented, lots of them playing since 4-5 years of age. I’m probably the worst performer in the bunch. Definitely the worst singer. “There’s a dying animal in the back” is a oft-occurring observation re: my singing skillz.

“So what do you do in music class?”

Parents, friends and family ask me this question. I start mumbling something incoherent as a response. We sing, we play chord sequences on the piano, we train our ears to recognize patterns (intervals, chords).

We also jot little dots on paper. This is to help us study music harmony in the style that Bach kinda invented. Or perfected.

Say you have a melody (like “Twinkle little star”). Imagine 4 people singing it at the same time, some lower, some higher. The four voices singing “Twin…” form a nice chord, hopefully. Then maybe a different chord when singing “…nkle” or “star”. Some of these chord sequences sound lovely. Especially when Bach did’em. We try to study the sequences that sound nice in an attempt to figure out why do they sound nice. And in an attempt to come up with our own. In studying we come up with rules of what sounds good, usually. Bach didn’t have these rules drilled into him, we don’t think. He did what sounded good to him. But we, as mini-Bachs, just taking off the ground, can use some rules. And maybe one day we’ll break’em!

We jot little dots on paper. Below is an example of my homework. The prof gives us the melody line and the chord sequence “recipe” and we fill-in the other 3 voices. Enjoy. (The prof found an error in the second assignment, can you?)

Some boring terms if you want to geek out, dig in, go off and head on into music theoryharmony:

• The 4 voices, from the highest to the lowest, are called soprano (the melody), alto, tenor, bass. Top 2 usually sang by women, bottom two by men
• Roman numeral analysis – staring into a piece of music for prolonged times and assigning numbers to its bits and pieces makes it easier to think of the piece on a higher meta level and not be bogged down with the actual notes
• Figured bass – the chord “recipe” you saw in the video. Dates back from Baroque times. Lets the performers have the freedom to fill in the blanks like I did in the homework. Except, you know, live. It takes me hours. Maybe one day…

OK, wtf is this?

Ugh, so many sharps. Eventually you learn them by heart. Like – but of course one sharp is G major. Also e minor. But to get there you need some tools. The circle of fifths is one such tool. But you need to move on from there. You don’t have time to draw a circle (even in your mind) every time.

So this post gives you two ideas to figure out key signatures without resorting to the circle.

Majors with sharps: raise half a step

Back to the example. What on earth can this possibly be? And let’s start with majors first.

You know how to traverse the circle of fifths and go F (forget it), C is 0, G is 1, D2, A3, E4, B5. Aha – B major!

But here’s a quicker way. Look at the last sharp. It’s A♯. Raise it by half step. Here’s your answer – B major.

Yup, that simple. Raise the last ♯ half a step. And bear the sharp in mind. You’re raising A♯ not A.

Wanna try another?

What is the last sharp? F♯. Raise it by half a step. G major. Boom!

Another? With all the sharps?

The last sharp is B♯ (same pitch as C). Raise is by half? C♯. There you go.

Nice, it works! How about the minors?

Minors with sharps: lower a step

A minor key has the same flats and sharps as the major which is a step and a half above it. A minor has 0 sharps and flats, just like C major. E minor has one sharp like G major. Theoretically if you know your majors you can derive the minor, just lower it a step and a half. But that’s doing two things. There’s a shortcut where you only do one thing. Fewer possible points of failure.

Take the last ♯ and lower it a whole step. Makes sense, right? For the majors you raise half a step and and the distance between a major and minor is a step and a half. 1.5 - 0.5 = 1

So let’s try.

F♯. Lower it a whole step – E. Answer: E minor.

Nice, gimme another!

The last sharp is B♯. Lower a step. A♯. Here’s your answer. A♯ minor.

Finally…

A♯ is the last. Down a step – G♯. G♯ minor – last and final answer.

Now go practice!

Let’s talk triads.

As the name suggests it’s a combination of three notes. The simplest chords are also triads, e.g. C major chord is the triad C, E, G.

Anyway, there are four types of triads – major, minor, augmented and diminished.

In this post you’ll learn how to populate a table of triads that is a helpful tool when starting with triads. This is the end result:

Now let’s see how to make sense of this table, how to populate it and how to use it going forward.

Intermission

This post is part of series where I spill the beans of what I learned in Music Theory 1 class from an awesome professor. He gave us these little tools and tables that suddenly made so much sense, more than anything I taught myself from various online and offline resources.

Other posts in the series:

• Circle of fifths
• Table of intervals

Tools to practice your newly acquired knowledge:

• Intervals
• Triads (topic of this post)
• Key signatures (apply the circle of circle of fifths)
• Note identification (you know, the very basics)

TL;DR

You already know intervals. Triads can be thought of as an extension of the intervals. Intervals deal with two notes (1 and 2). Triads deal with three (1, 2 and 3). The relationship between notes 2 and 3 is also an interval.

If you only think of the intervals between consecutive notes, then all you need to remember is where does major third go and where does minor third go. Simple. Only 3rds. Only Major and minor 3rds. Nothing else. Don’t you worry about 5ths. Forget scale degrees.

Depending on the intervals between notes 1, 2, and 3 you have:

• Major triad. Note 1 to note 2 is a major 3rd, note 2 to note 3 is a minor third.
• Minor triad. The reverse. 1 to 2 is minor third, 2 to 3 is major third
• Diminished. Both intervals are minor thirds.
• Augmented. Both intervals are major thirds.

Back to the scheduled program. Triads.

Here’s a scale. The simplest. C major. All white keys on the piano.

Number these notes. Call them “degrees” in the scale:

You only care about up to the 5th when it comes to triads:

And not even all five, just 1, 3, and 5:

Ignore all but these three (triad!) notes in the scale.

Look at the intervals between these. C to E is a major third. E to G is a minor third. (If this makes no sense, go back to the post about intervals)

Now let’s start populating out little table of triads.

The first entry means: a major triad (also commonly spelled “M”) consist of three notes where the interval between the first and the second is a major third and the interval between the second and the third is a minor third.

These three notes also happen to be the natural 1, 3 and 5 degrees of the major scale.

Coming up next – the minor triad. What could it be?

Oh by the way let’s populate the last column with 1, 3, 5. All triads use these three (triads!) just some of them move a little.

Now back to the minor triad.

The minor triad is created by lowering the third.

In other words, on our C, E, G example, the third E becomes E♭.

Look at the new intervals. 1 to 2 is a minor third, 2 to 3 is a major third.

We can put this new information into the table and move on with our lives.

Moving on. With our lives. What’s next? Diminished.

The diminished triad consists of two minor thirds. Is all. Don’t ask me why. That’s just the way it is. Diminished (small) consist of two of the smaller kind of 3rds (where minor is smaller and major is bigger).

In terms of scale degrees, you lower the third and lower the fifth as well.

Here comes the staff. Two minor 3rd intervals:

Last ‘un: augmented triad. Also commonly written as Aug. Capital Aug. Lowercase aug. Capital M. Lowercase m.

So, augmented. What could it be? Sounds big. Which was the bigger 3rd – major or minor? Major, that’s right.

The augmented triad has a major third between notes 1 and 2. And another major third between 2 and 3.

When it comes to scale degrees, you leave 3 be (it’s already major) and raise the fifth so the second interval becomes major.

That’s it, now you have populated the whole table of triads. Ain’t that cool. Look at it, just look. At. It.

Examples

Let’s see a couple of other examples. Cause, you know, not all triads start from C.

Let’s start with G and draw two more circles (pancakes) above it. That would be B and D.

G to B is major 3rd. (M!) B to D is a minor third. So M-m. A major triad. M. Cool. Then lowering the 3rd (by way of a ♭) gives you a minor triad (cause 1 to 2 is minor and 2 to 3 is major). Then lowering both the 3rd and the 5th gives you a diminished triad.

Finally restoring the 3rd where it was (major) and augmenting the fifth gives you two majors. And M and a M means two majors, in other words an augmented tried. Enjoy!

One more example. Draw an A and two more pancakes (notes) above it. What do you have? A, C, E. ACE. A to C is a minor third. C to E is a major third. So m then M is a minor triad.

Upping the third (with a ♯) gives you A to C♯ (a major third) then C♯ to E – a minor third. Looking back at the table you can see that M followed by an m is a major third.

Augmenting the 3rd note in the triad (which happens to be the 5th in the scale) gives you an M followed by an M. Two Ms is an augmented triad.

Bringing down the C where it naturally was, gives you a minor third (A to C), and lowering the E to E♭ means C to E♭ is another minor third. Two minors spell a diminished third triad. Enjoy!

That’s all, folks!

Now go practice!

In the previous installment you learnt how to draw this table of intervals:

Now let’s figure out how to use it and also finish it up until it looks something like:

OK, let’s start by figuring what in the world is this interval supposed to be:

This is C and G. Let’s count the number of lines and spaces, starting with the initial note.

There are 5 lines and spaces. Same if you use the alphabet ABCDEFG and count C = 1, D = 2, E, F, G = 5.

You can also use the little helper tool and note the number next to G.

At this point we’re pretty clear these are five lines and spaces. Write it down.

Now you need to count how many semitones are between C and G. Using your keyboard, put your finger on C and count every time you move. You end up counting to 7.

So 5 lines/spaces and 7 semitones.

Time to look at the table of intervals. You find a row that says 5 (# lines/spaces) and 7 (distance in semitones). We have a match!

Therefore you conclude that the interval C to G is a perfect fifth. Success!

Now what if you have G♯ instead of G. What do you call the interval between C and G♯? G♯ is still on the same line. So the number of lines and spaces is still 5.

But what about the semitone distance?

Using the keyboard to count you end up with 8.

But in the table we don’t have 8. We have 7.

The interval is no longer perfect, because it was augmented with 1. Hm, what do we call it? Here’s an idea – we call it augmented fifth.

In conclusion, the interval between C and G♯ is called augmented fifth.

Now what if you have G♭ instead of G? Same 5 lines of spaces but different semitone number.

Using the keyboard and counting, you get to 6. (Starting from and skipping C, you have C♯ = 1, D = 2, D♯ = 3, E = 4, F = 5, G♭ = 6).

We don’t have a match in the table for 5 lines/spaces and 6 semitones. We have 5 lines and spaces, so it’s a fifth. Only the perfect 7 was diminished by one. How do we call this? You guessed correct – a diminished fifth.

In conclusion, the interval C-G♭ is called diminished fifth.

Let’s add this new information to the table: every time you add 1 to a perfect interval, you call it augmented and every time you subtract 1 you call it diminished.

Things are similar, yet a little different, when it comes to the intervals in the second column, the group #2 where the major intervals live.

Adding one still produces an augmented interval. Subtracting 1 is called a minor. Subtracting 2 is now called diminished.

So there you go – the last and final version of the table of intervals. Using this you can figure out any interval there is.

Let’s practice, this time with the major side of the table.

What is this? E to G.

Counting lines and spaces (including the starting point) gives you 3.

So it’s a third interval.

You find the third on the major side of things. It asks for 4 semitones. Is this what we have?

Let’s count on the keyboard. Put a finger on E and count every time you move. F = 1, F♯ = 2, G = 3.

So we counted 3 lines/spaces and 3 semitones.

The table asks for 4 semitones. But we have 3.

Looking at the bottom of the table for the short list of corrections.

You need to subtract 1 to get a 3 from a 4. So turns out the interval is minor.

To sum it up, the Answer to the question what is the name of the interval between E and G… it’s a minor third.

What about E to G♯? It’s still a third (3 lines and spaces on the staff) but with the 4 semitones it matches the number in the major pat of the table. So the interval between E and G♯ is a major third.

What about the interval E to G♭. Still a third (3 lines/spaces) but with 2 semitones in between (4 – 2 = 2). It’s diminished. All in all the interval E to G♭ is a diminished third.

And what about E to G-double-sharp? Again a third, but with 5 semitones between them (4 + 1 = 5), it’s an augmented third.

And this is how we counted the semitones…

E to G♭… F = 1, F♯ (aka G♭) = 2. Diminished third (because the regular major third asks for 4 semitones and major – 2 = diminished).

E to G. F = 1, F♯ = 3, G = 3. Minor third. (Major – 1).

E to G♯. F = 1, F♯ = 2, G = 3, G♯ = 4. Major third (a match in the table)

E to G-double-sharp (Gx is also A). F = 1, F♯ = 2, G = 3. G♯ = 4, A = 5. Augmented third.

Wrapping up

There you have it, ladies and gentlemen. A table of intervals that gives you a way to figure out any interval.

Thank you for reading. Now go practice. Write down a random thing on the staff and go figure it out.

Here’s a start. C to A♭. We did C to G♯ above, and it’s the same sounds. But because it’s written differently, the interval has a different name. What is it?

OK, here’s some more random stuff I put on the staff for you to practice:

OK, now don’t scroll any more, before you’ve done these yourself.

Because I’ll give you the answers.

OK.

Got it?

No more scrolling.

Here come the answers now.

Stop scrolling.

Stop it!

I mean it!

Knock it off!

Enough!

Yo!?!

BTW, If I got any of the answers wrong, please tell me I’m an idiot, publicly on Twitter. (Shaming works miracles!) So I can correct it for the future readers who come across this page 104 years from now. Think of the children.

K, answers:

1. fourth, perfect
2. fourth, augmented *
3. fifth, perfect
4. second, major
5. second, minor
6. fourth, perfect
7. fifth, diminished
8. third, minor
9. fifth, perfect
10. fifth, perfect
11. third, diminished
12. fourth, augmented
13. third, diminished
14. second, minor
15. fourth, perfect
16. octave, perfect

* Augmented fourth (or diminished fifth) is the devil’s interval. It was banned by the church at some point as it sound jarring. And how can you blame the ole priest – if you play this interval on an organ in a echoey church, it’s not that pleasant. Nowadays we have our ears more sophisticated, we can appreciate weird stuff like this. Jazz. Blue notes. If played in passing and separately they are cool. If played together as part of a heavy metal composition – hell, yeah! The devil! It’s tricky though. Distorted guitars like perfect fifths. And octaves. Unisons. Maybe, perfect fourths if not too distorted. Playing diminished fifth on a distorted guitar requires them skillz.

Hello and welcome to this 2-part blog post on intervals. I mean intervals such as “major third” and “augmented fifth”, things like this. Extremely important building block in Music Theory. You need intervals to transpose stuff for example. Or, much more importantly, to figure out how to make chords (triads or more complicated ones).

Eventually with practice you should have these intervals memorized but to get off the ground you need some help.

(psst, do you want to learn about the Circle of fifths too?)

Tl;DR

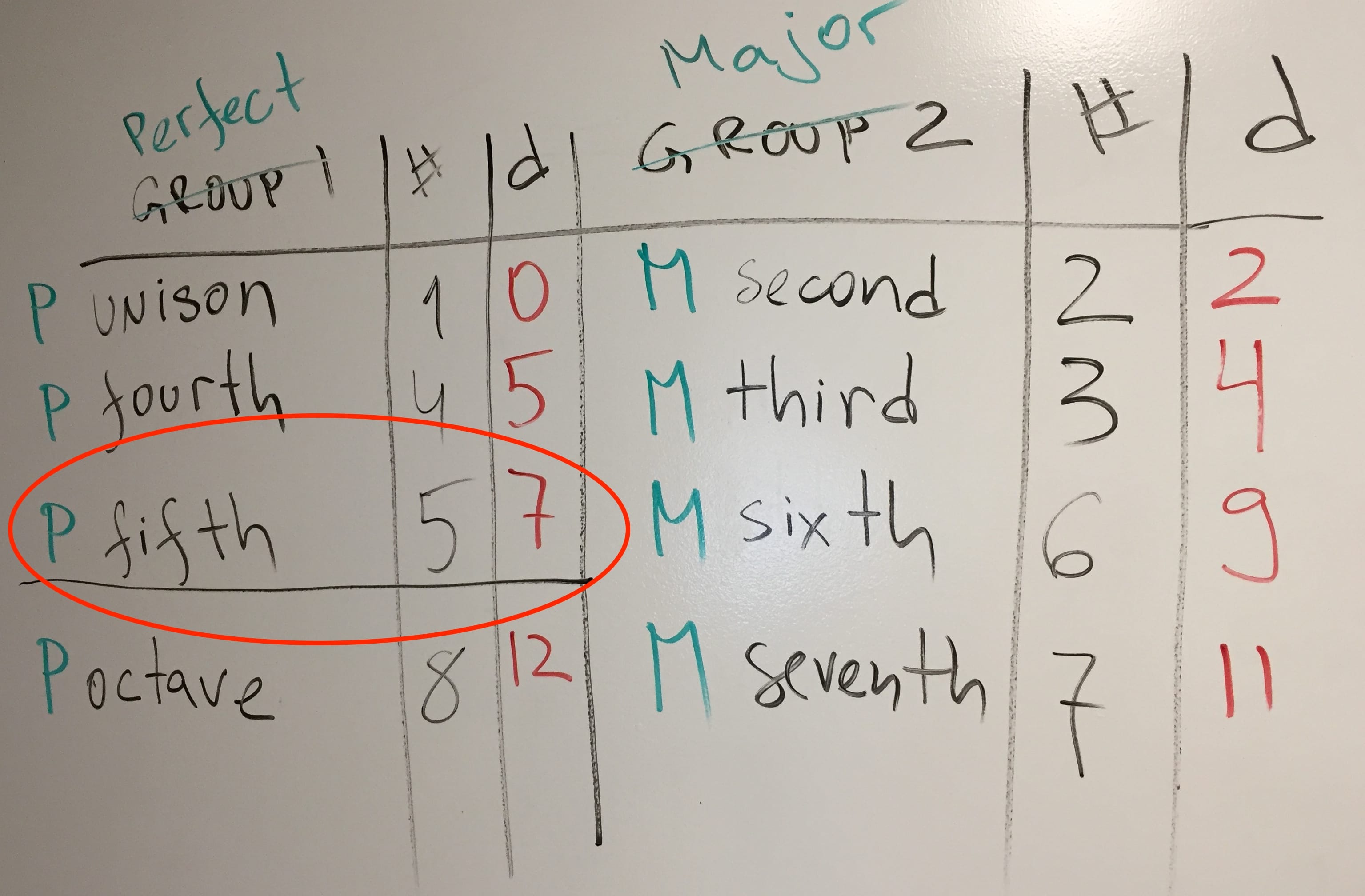
Starting with the end in mind, here’s all you need in order to figure out any interval ever:

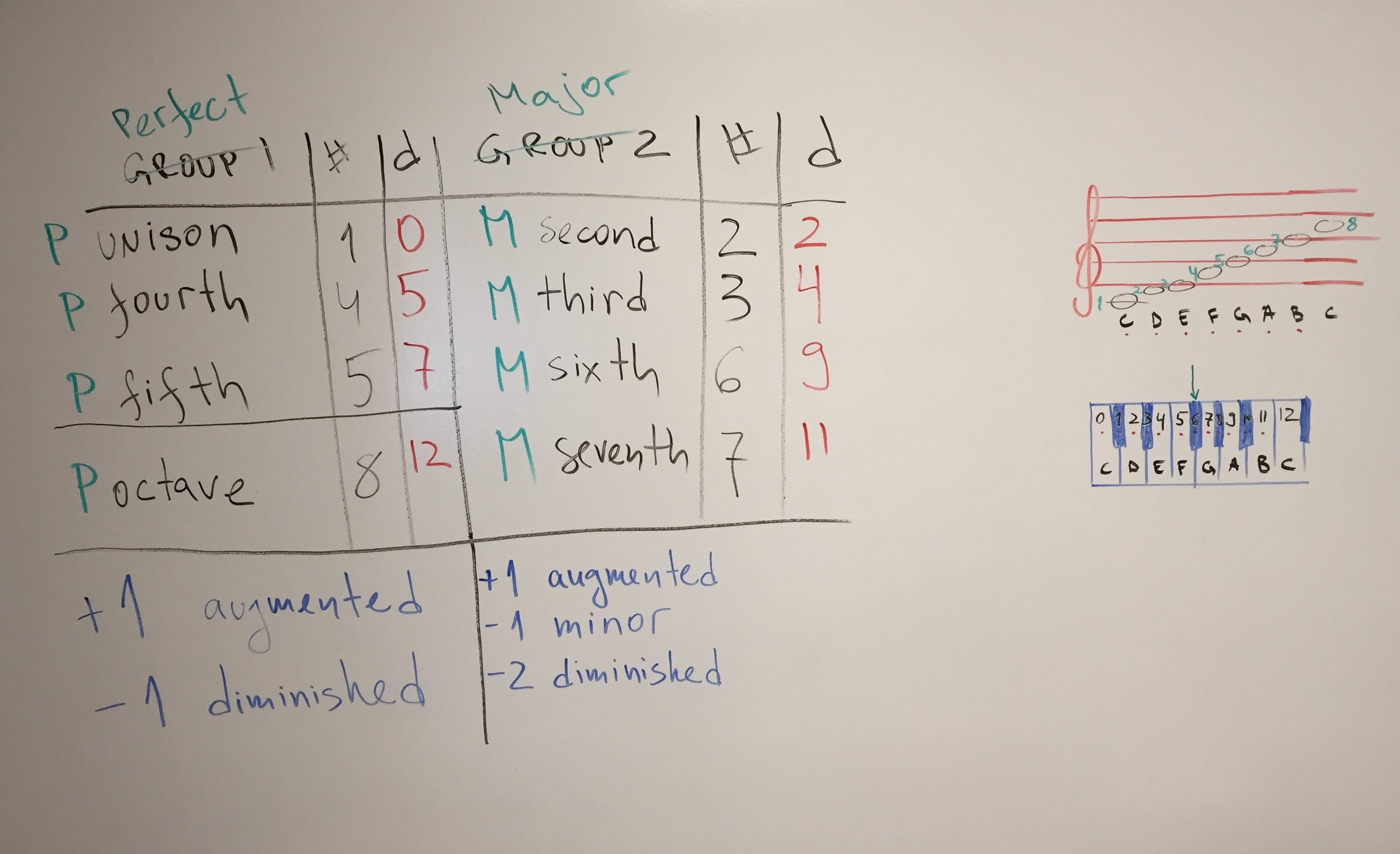
In this first post you’ll learn how to draw most of this table, in the second – how to finish and use it.

You should be able to draw this on your own so you don’t rely on referring to a piece of paper (that you lost last week, most likely) and also so that you memorize the intervals sooner. DIY FTW!

Mother’s little helpers

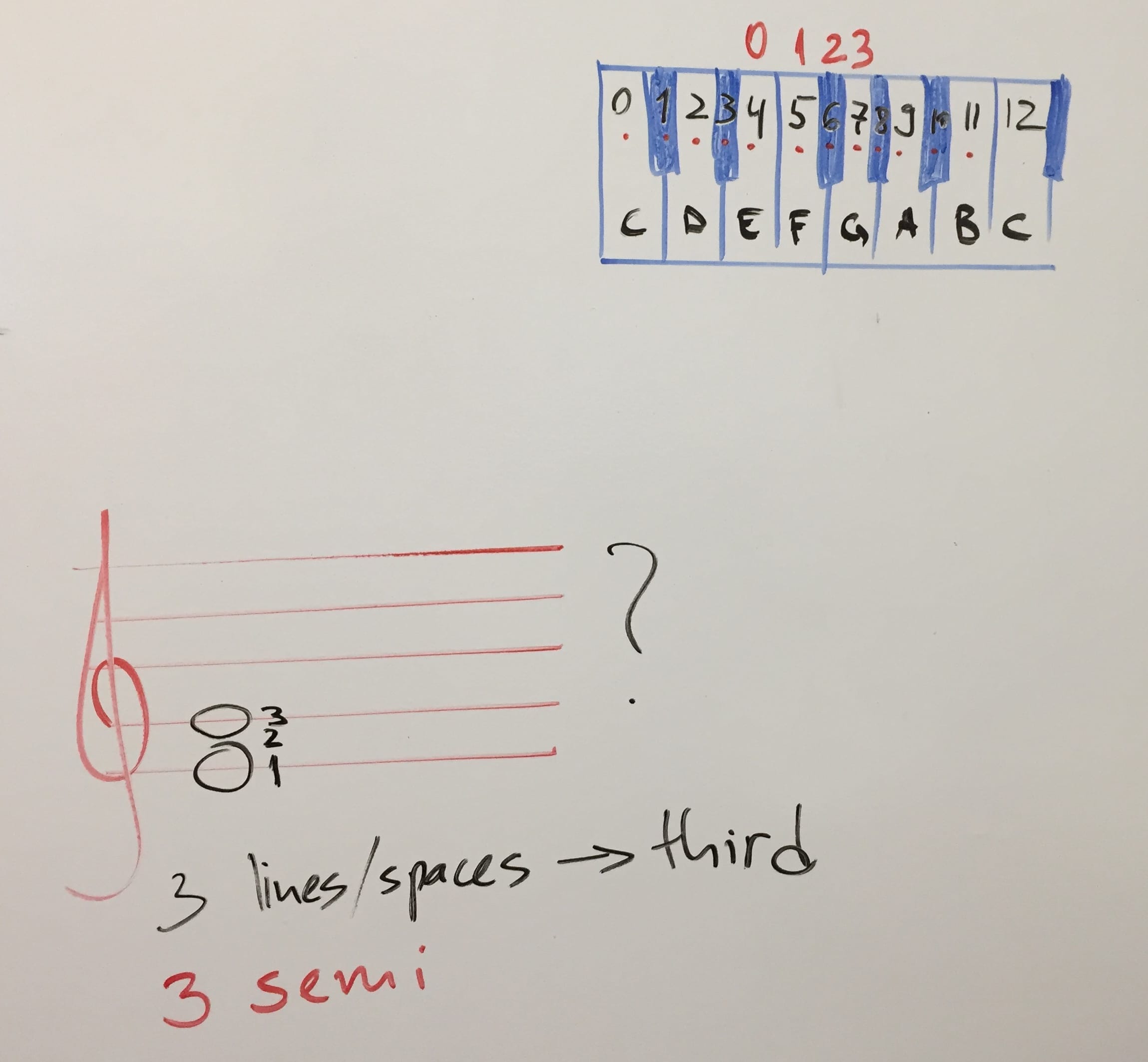
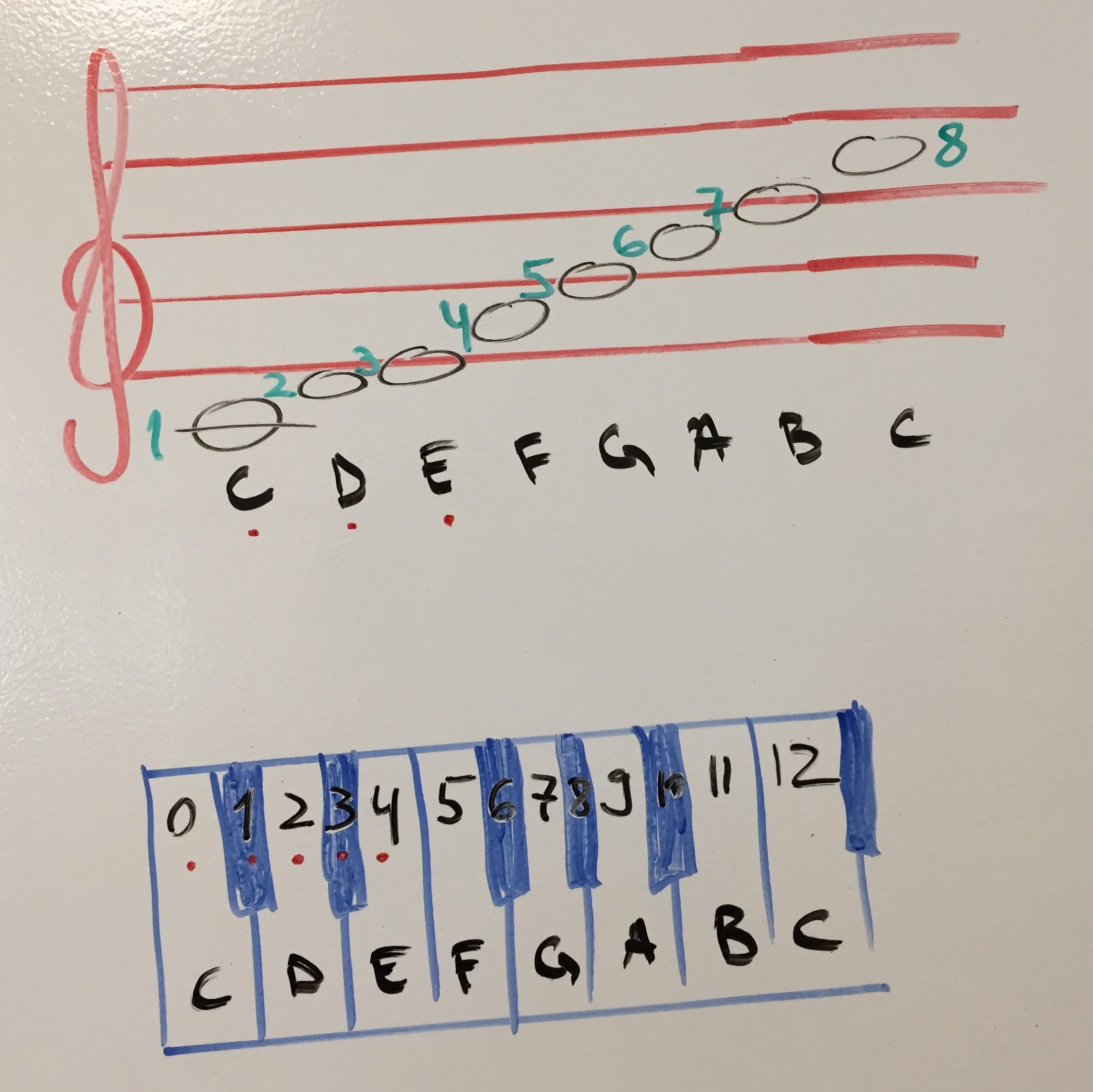
You’ll need some aids. The first one is the C major scale. Pancakes all the way!

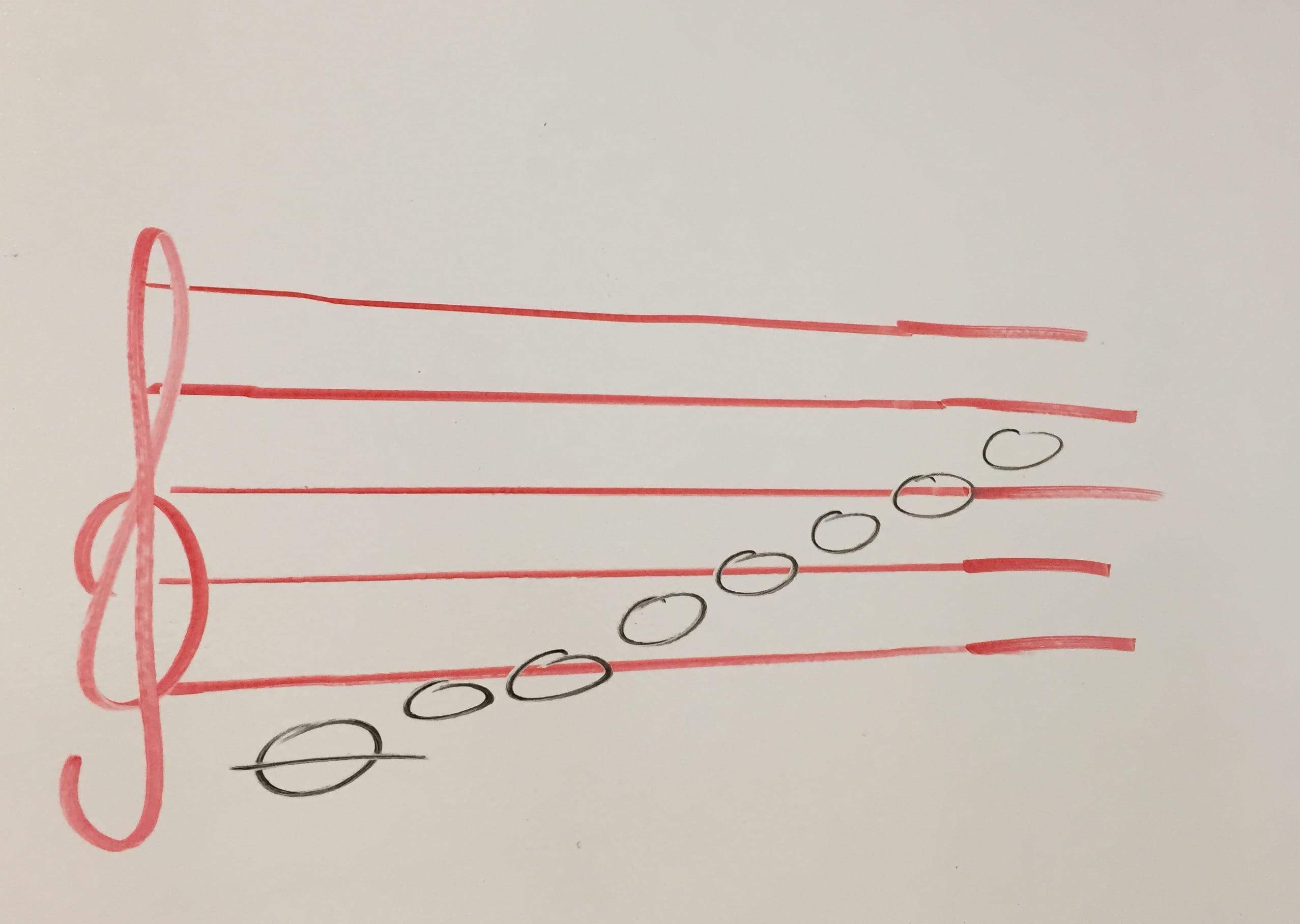
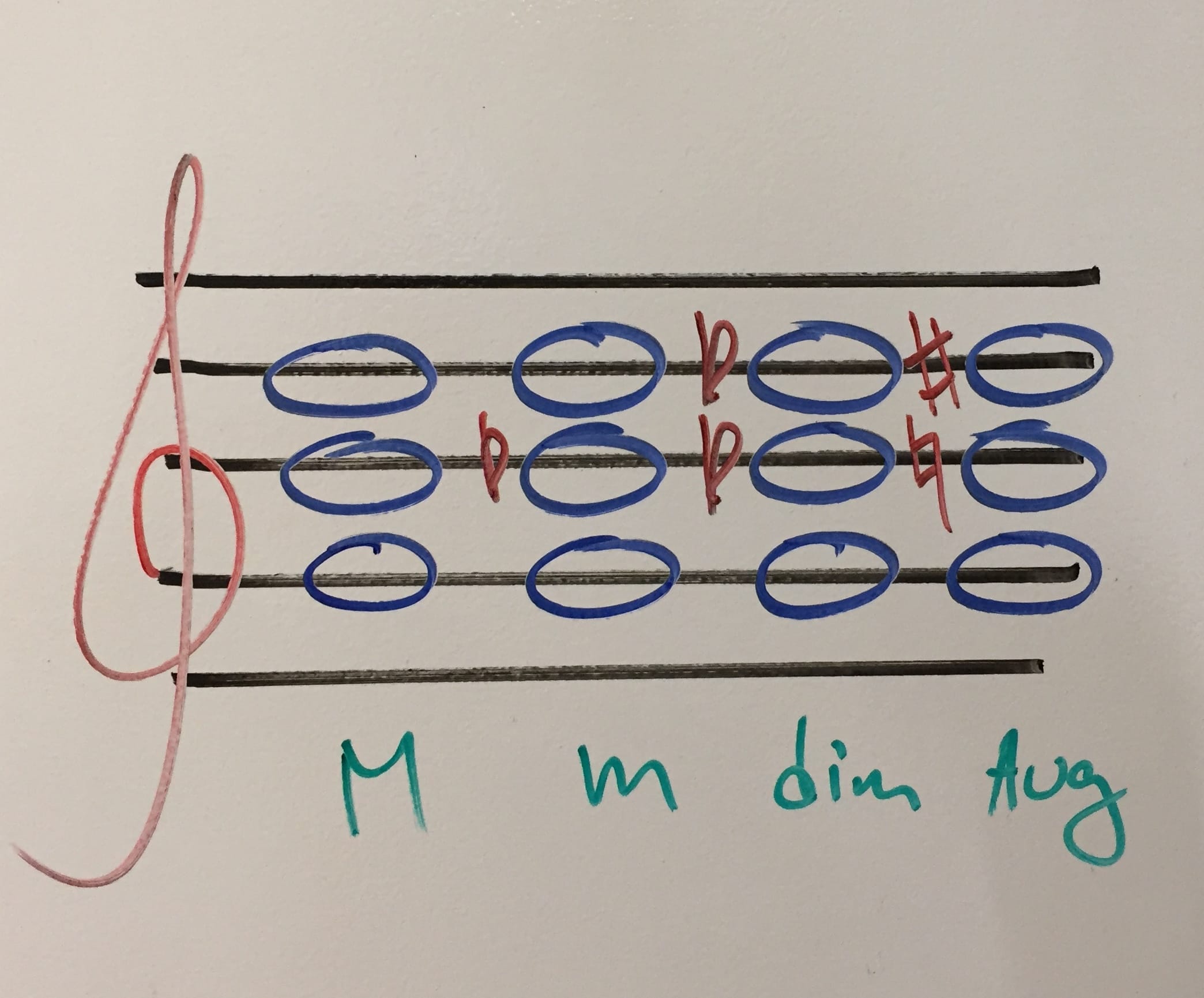
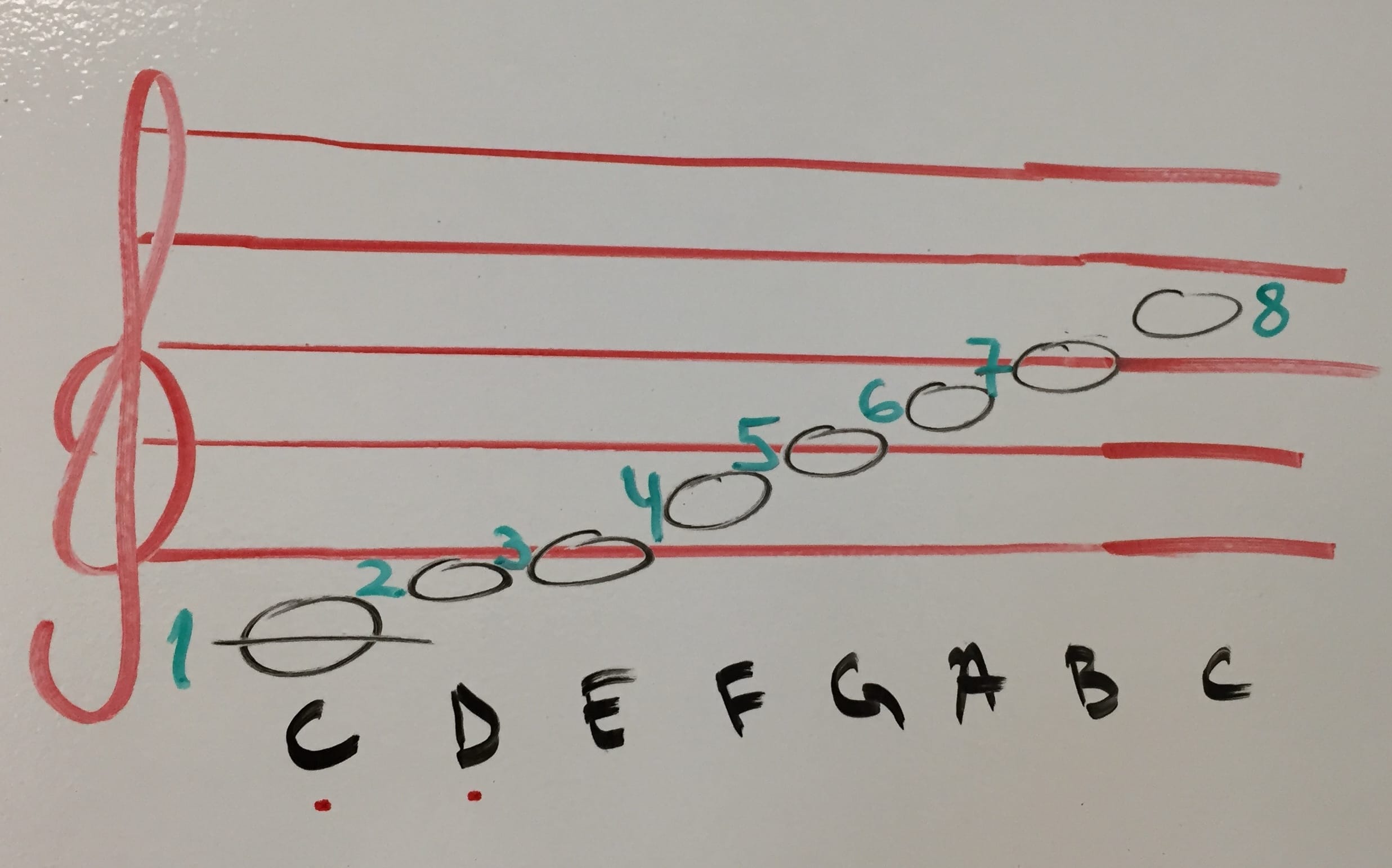
From C to shiny C:

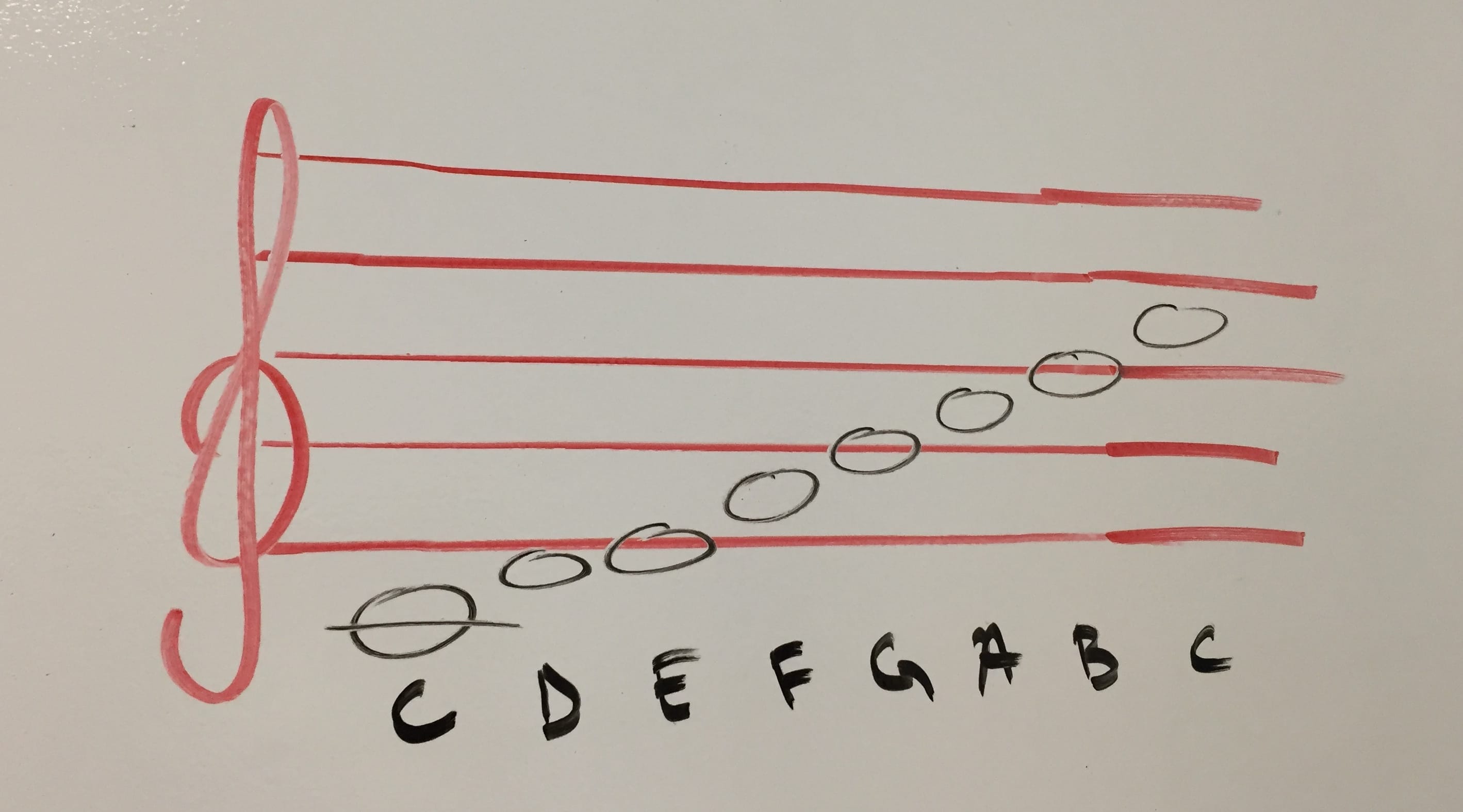
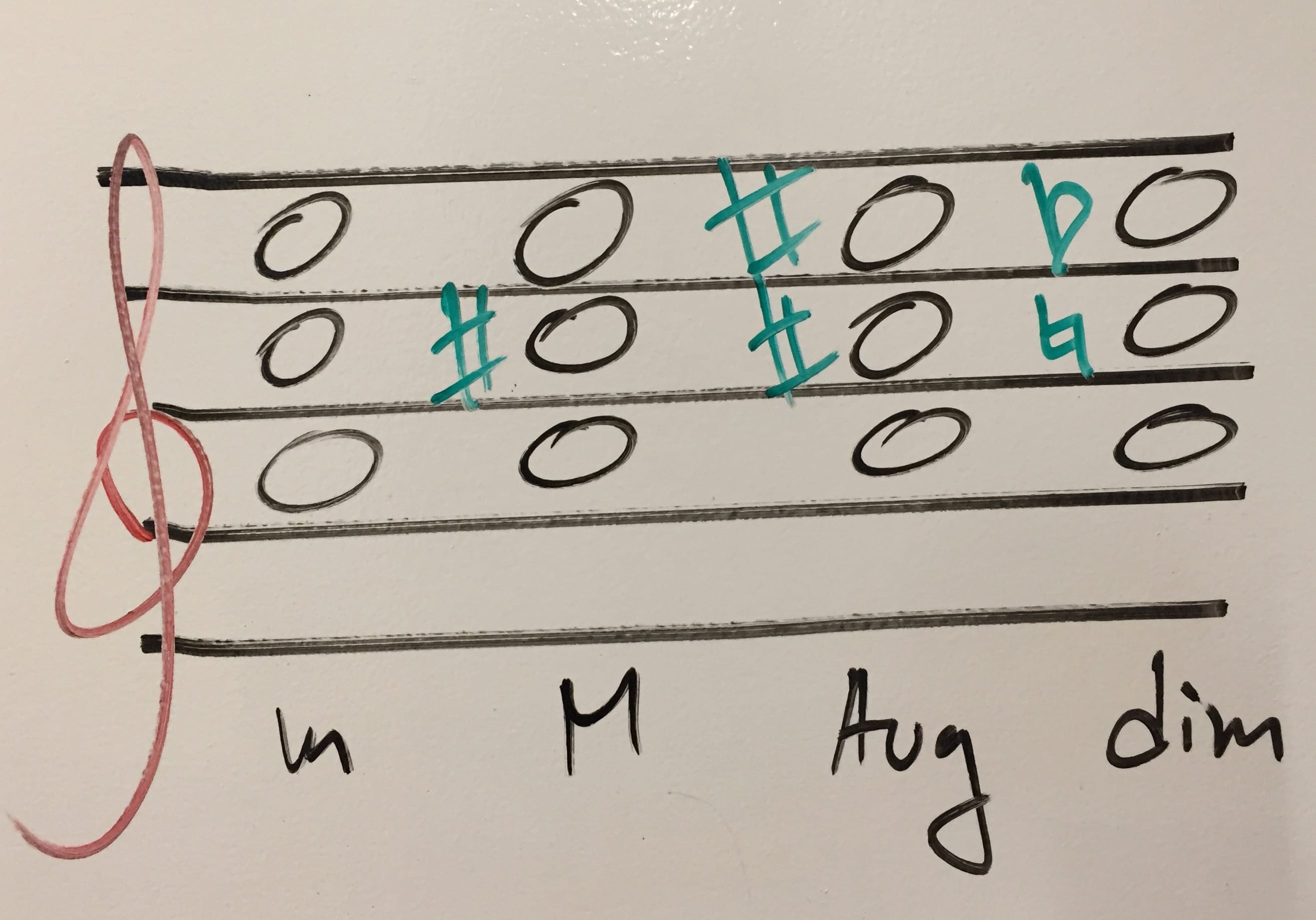
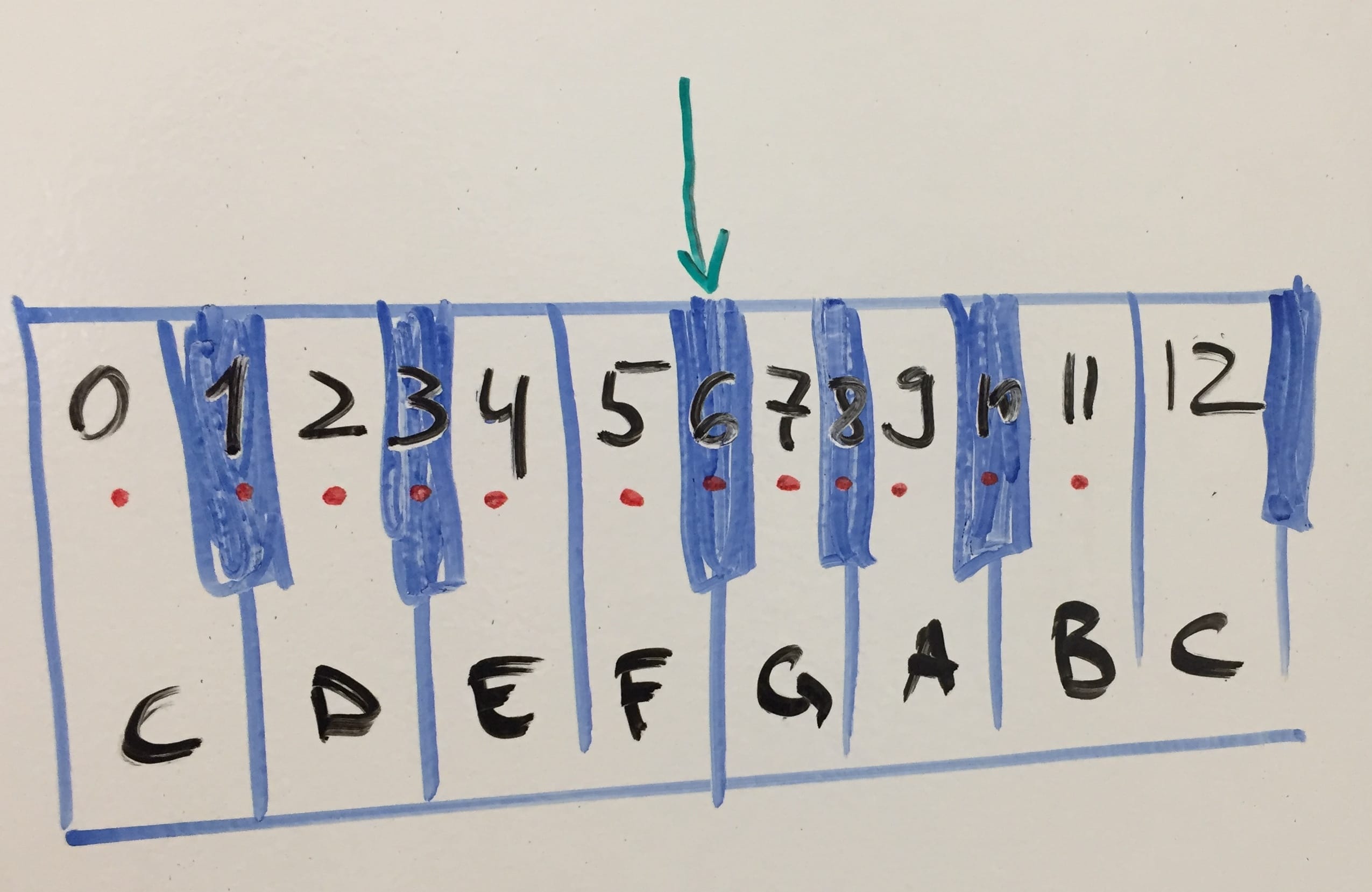
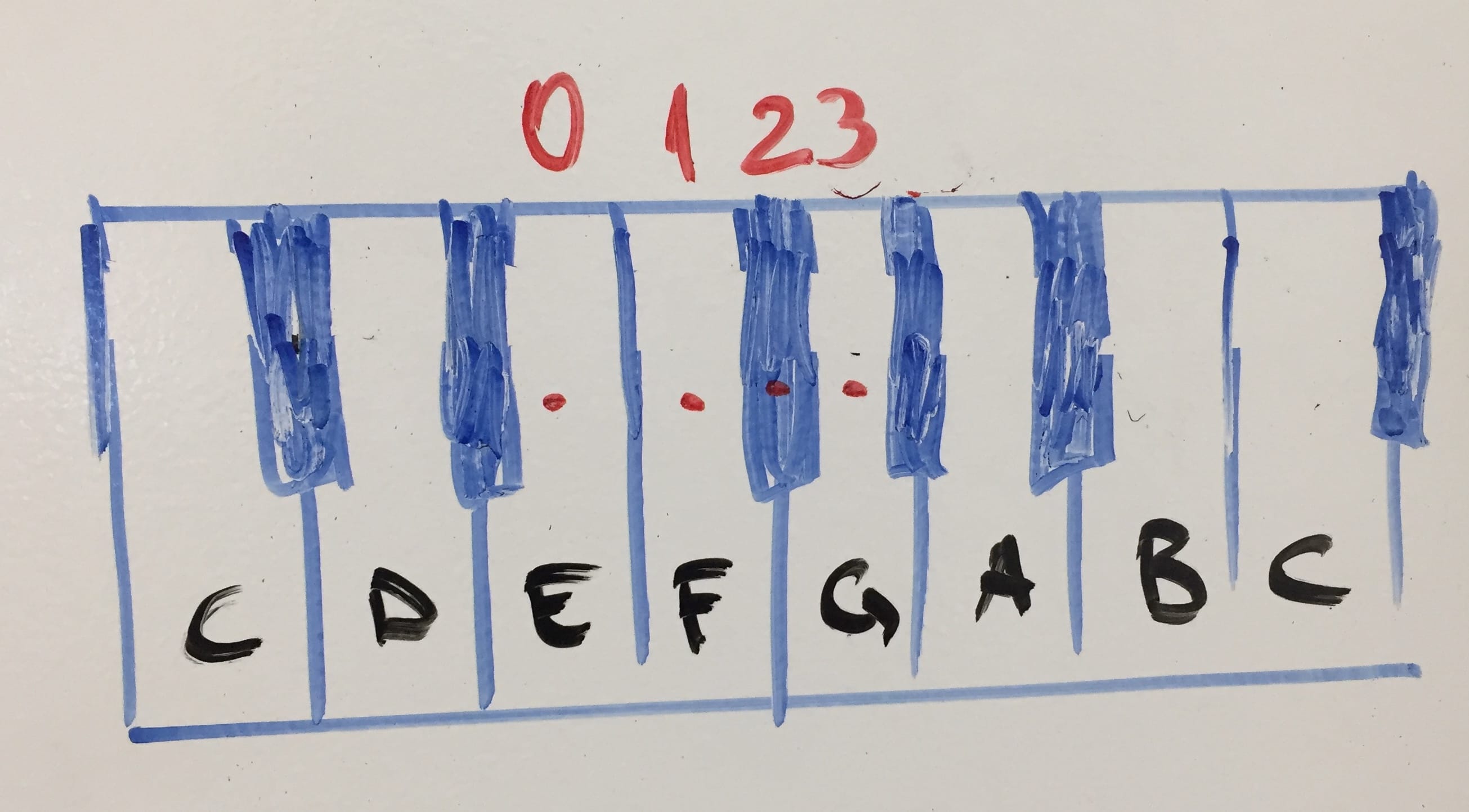
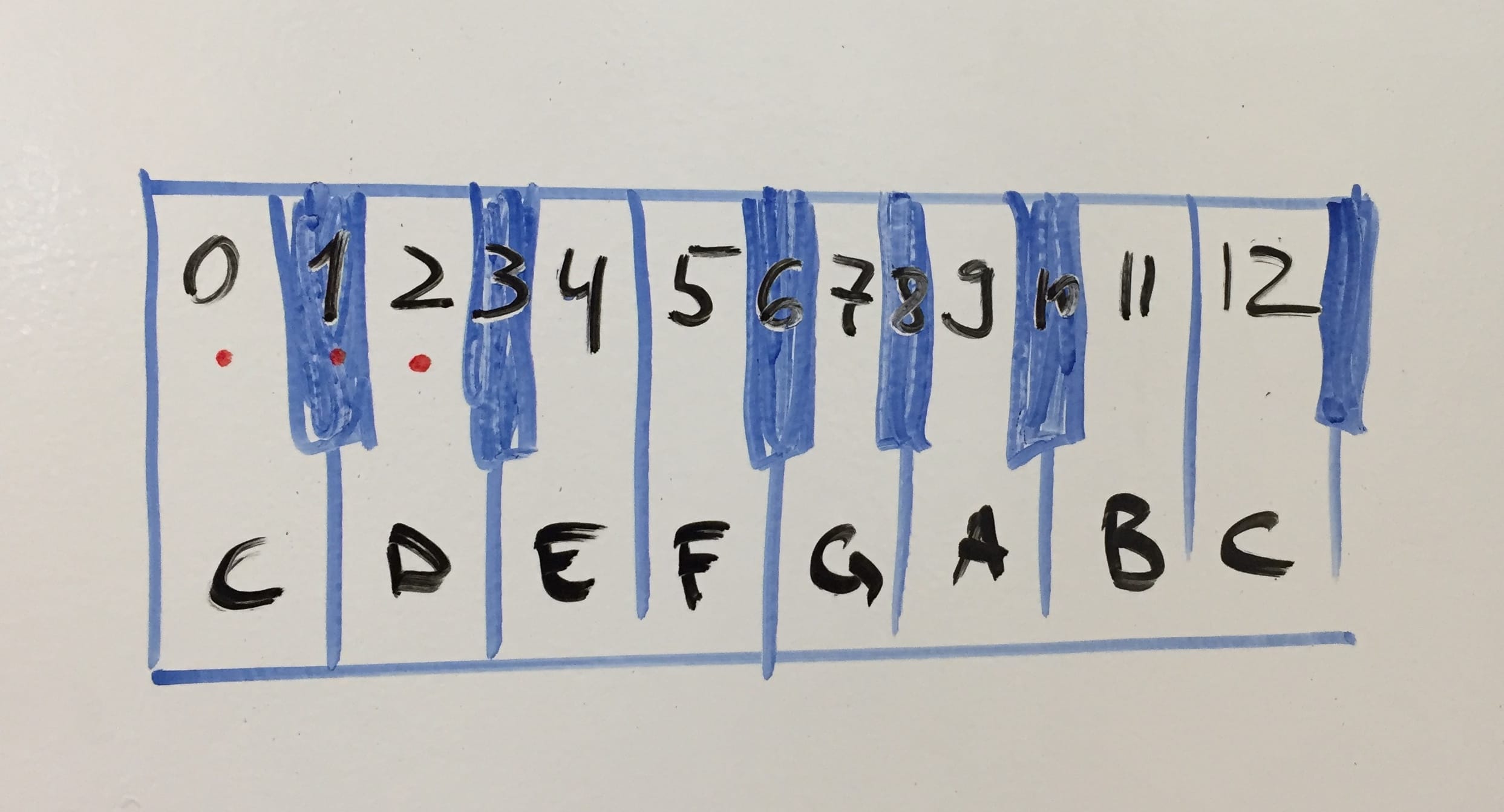
Next, you need a keyboard. You know how to draw a keyboard, right? Rectangles for the white keys, then the black keys go in groups of 2, nothing, 3, nothing, 2, nothing, 3, nothing… Finally the white key next to the group of 2 (that look like Chopsticks) is C. The white key next to the cluster of 3 (that look like a Fork) is F. Chopsticks – C, Fork – F. Fill in the rest using your mad alphabet skills.

So there – these are the visual aids: a staff with C major and a keyboard:

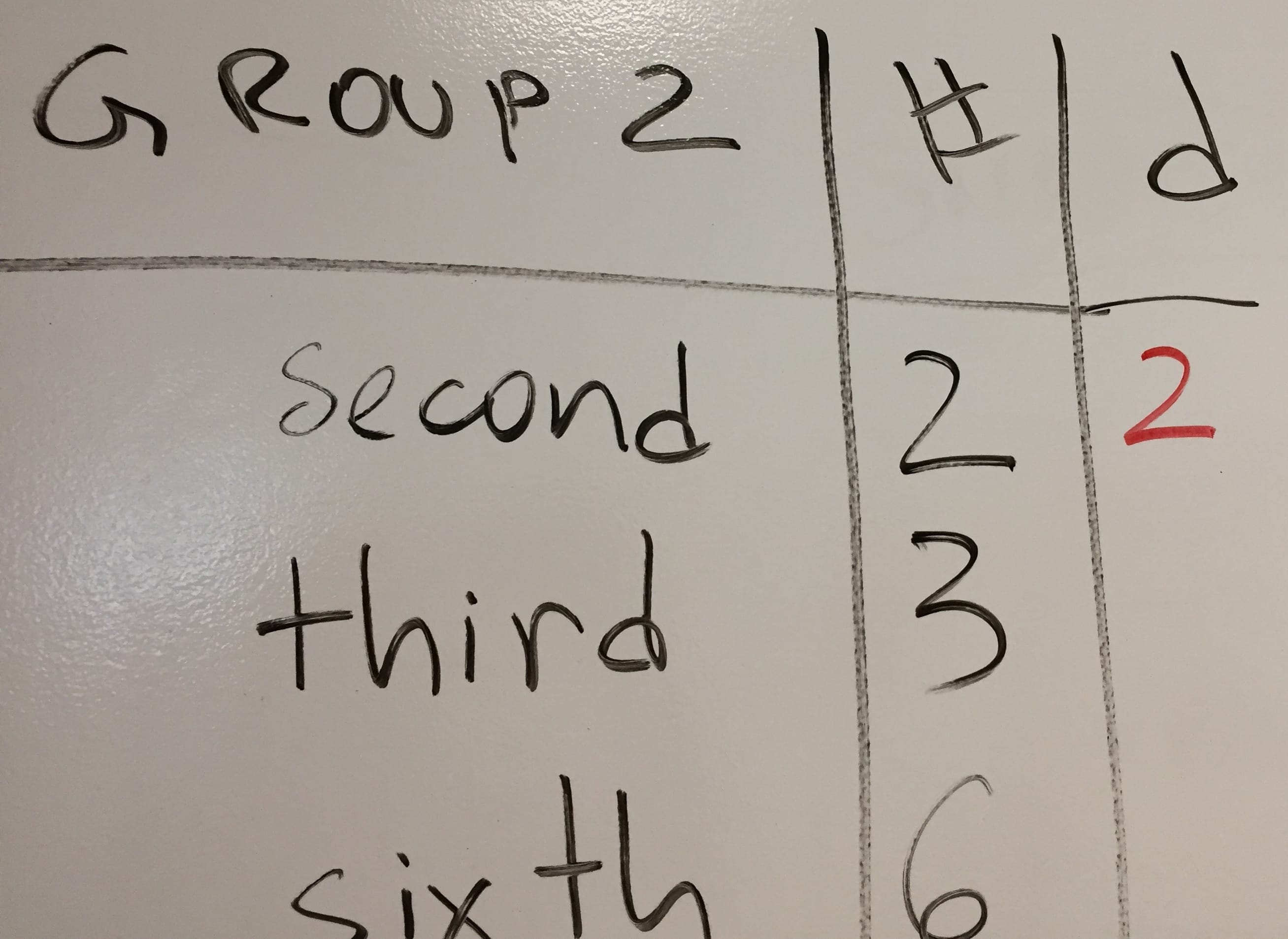
The table of intervals

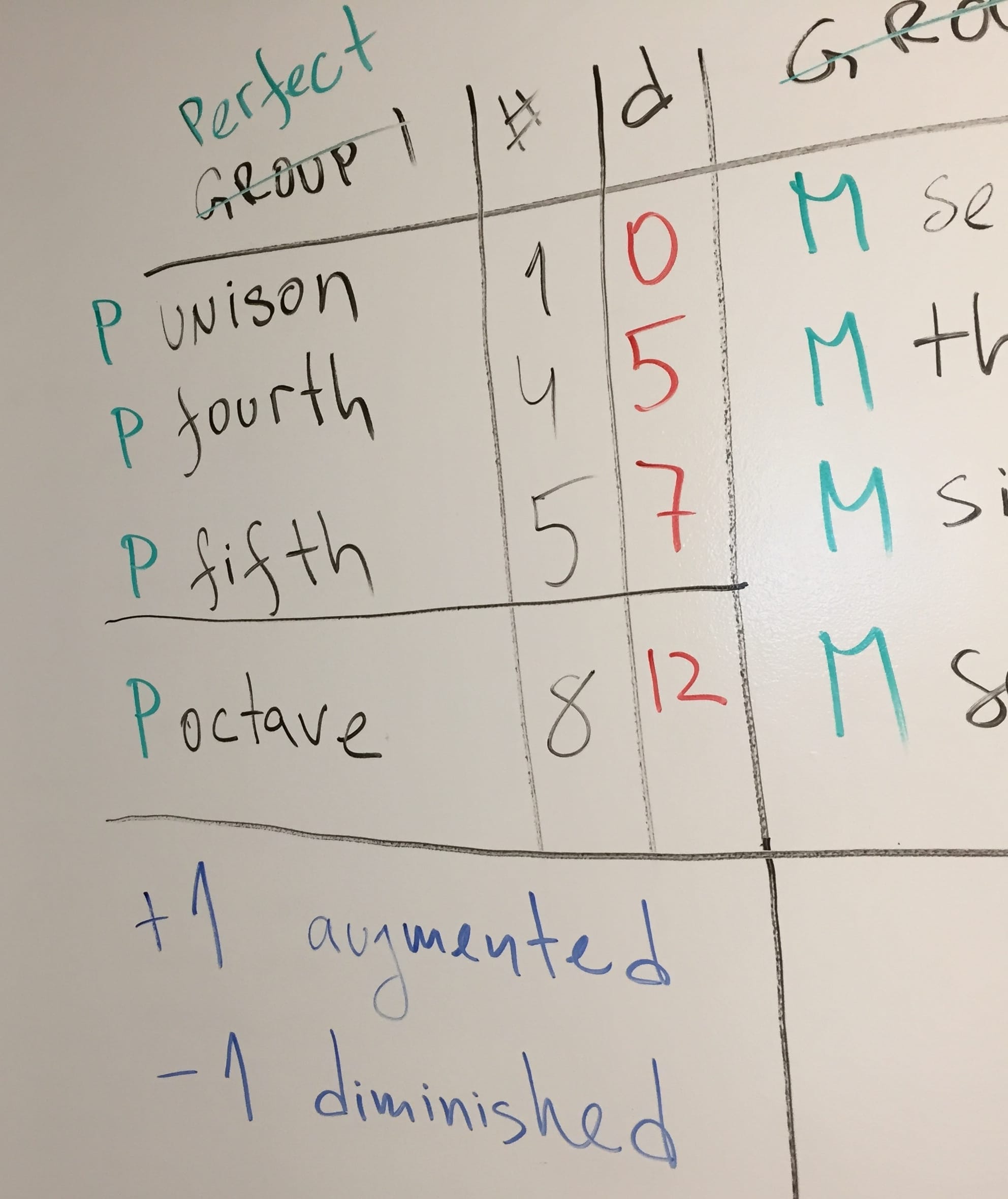
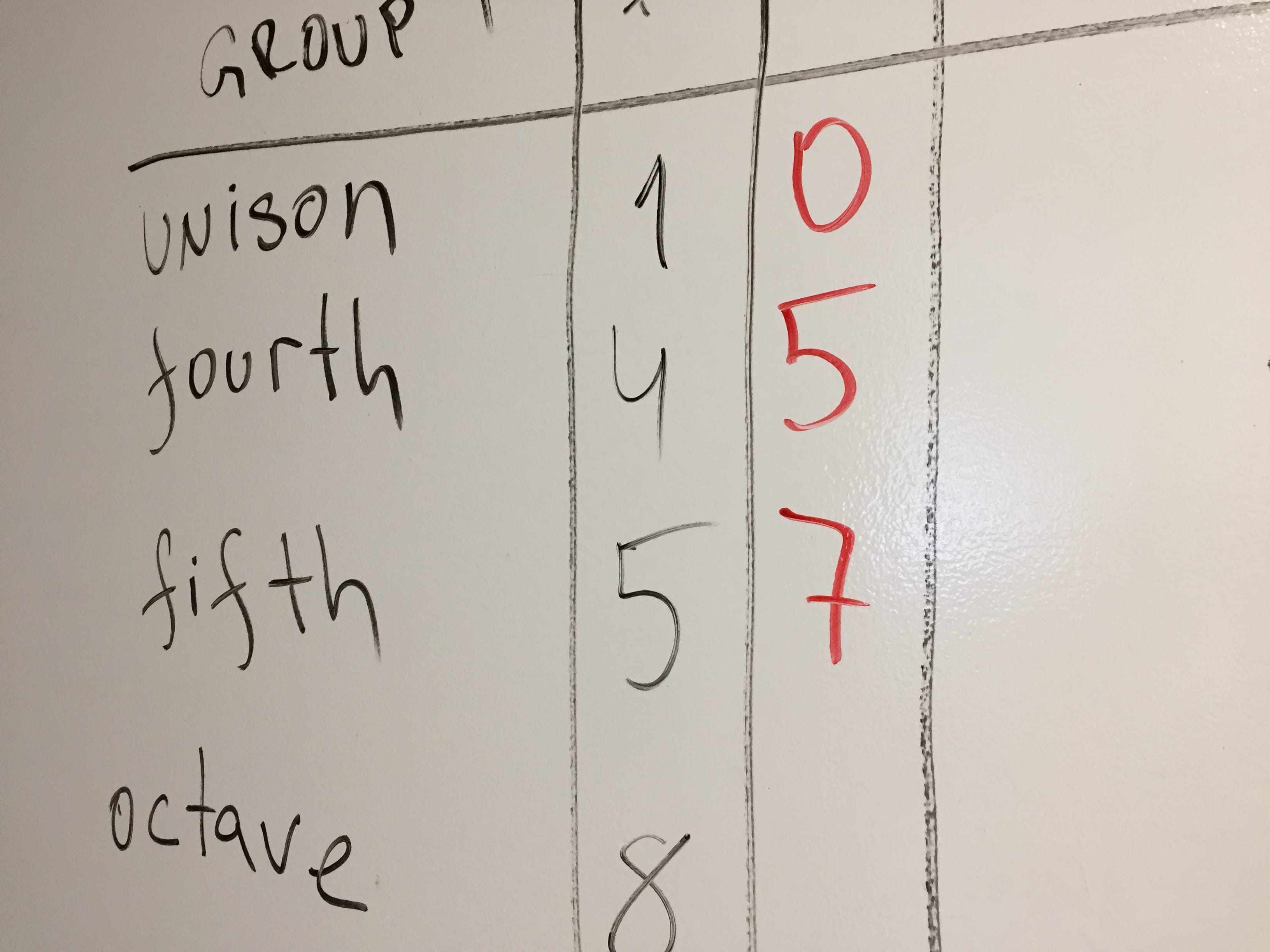
Time for the table. It starts with two groups. The intervals belong to one of the two groups.

Each group gets two more columns: “#” for “number of lines and spaces on the staff” and “d” for distance in terms of semitones on the keyboard.

Now you need to put the numbers 1 through 8 in the # column. 1 goes first on top left.

2 is top right.

3 goes under 2.

4 goes to the left of 3.

5 is under 4.

6 is to the right of 5.

7 under 6.

And finally 8 is bottom left.

Did you see a pattern? It’s a snake!

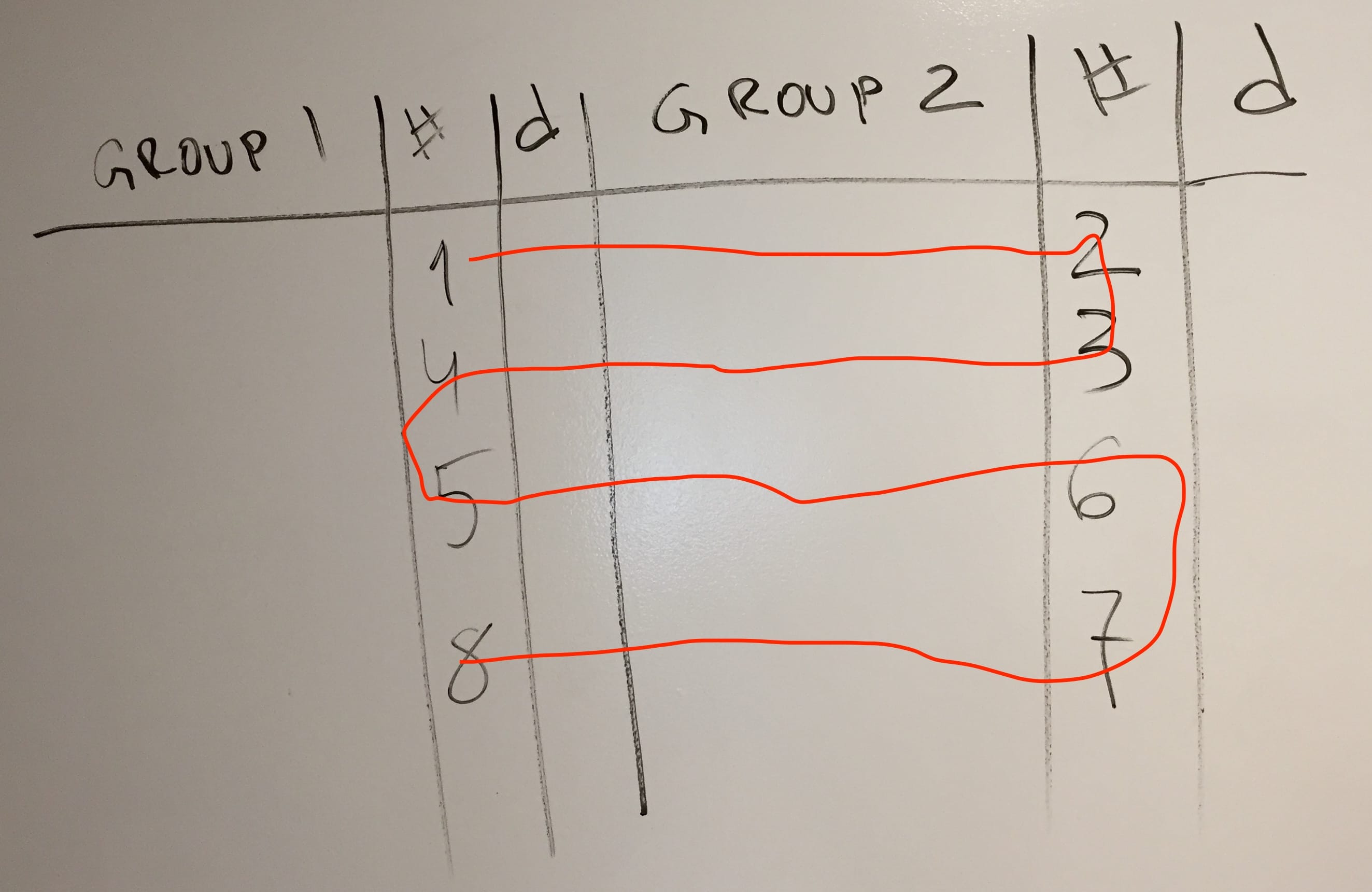
Why 8 do you ask? 8 is the number of lines and spaces between C and C. More on that later.

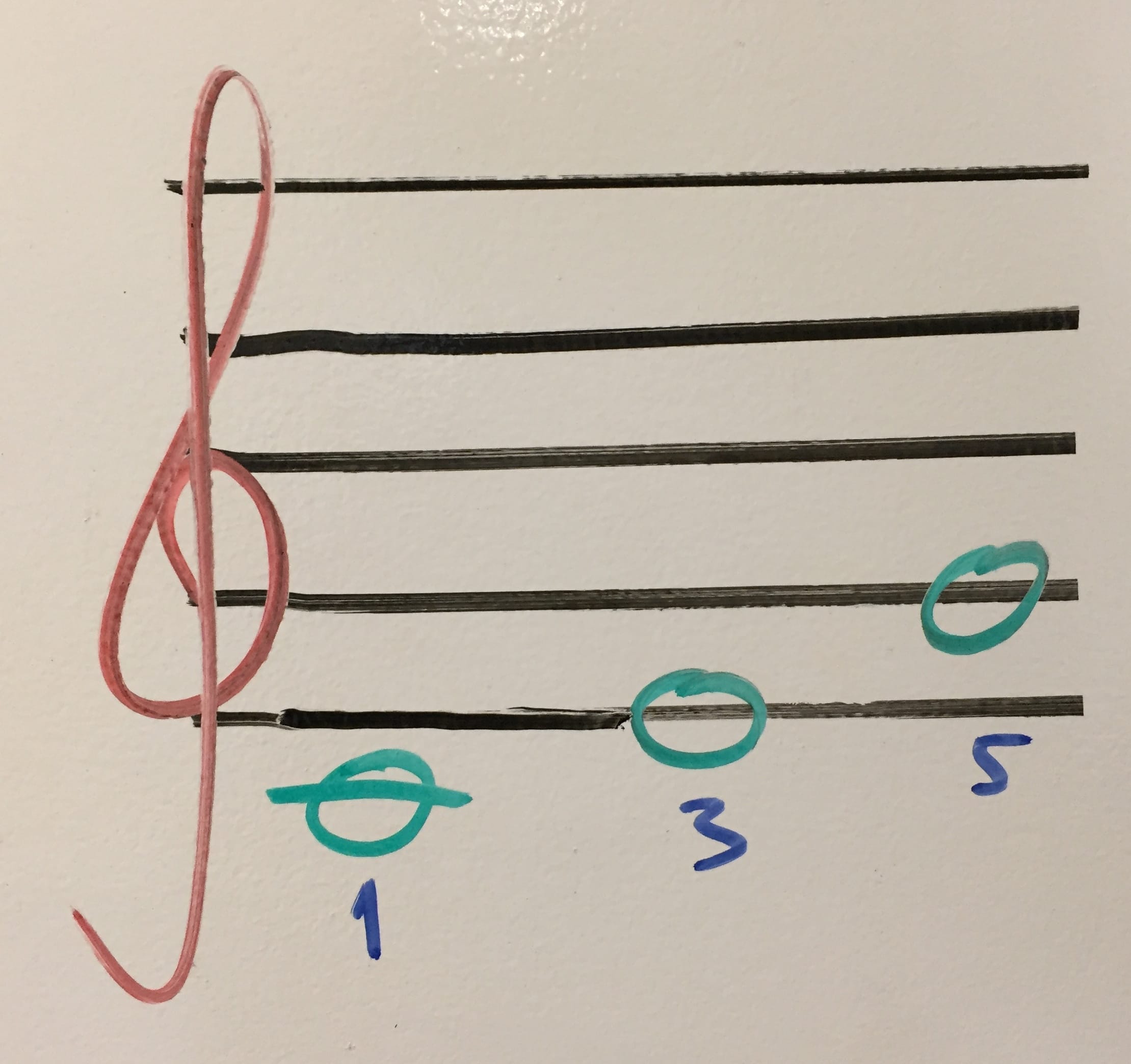
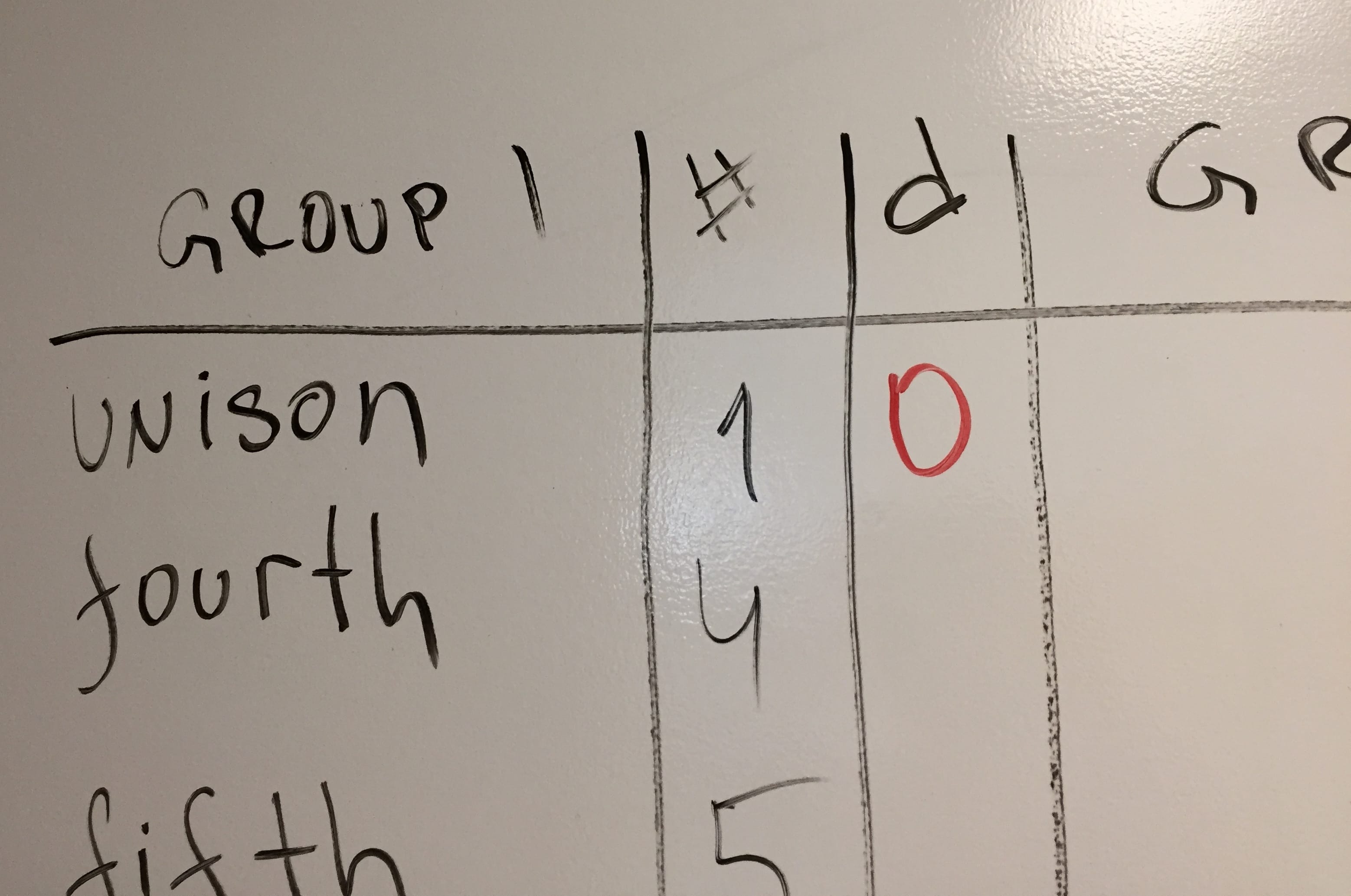
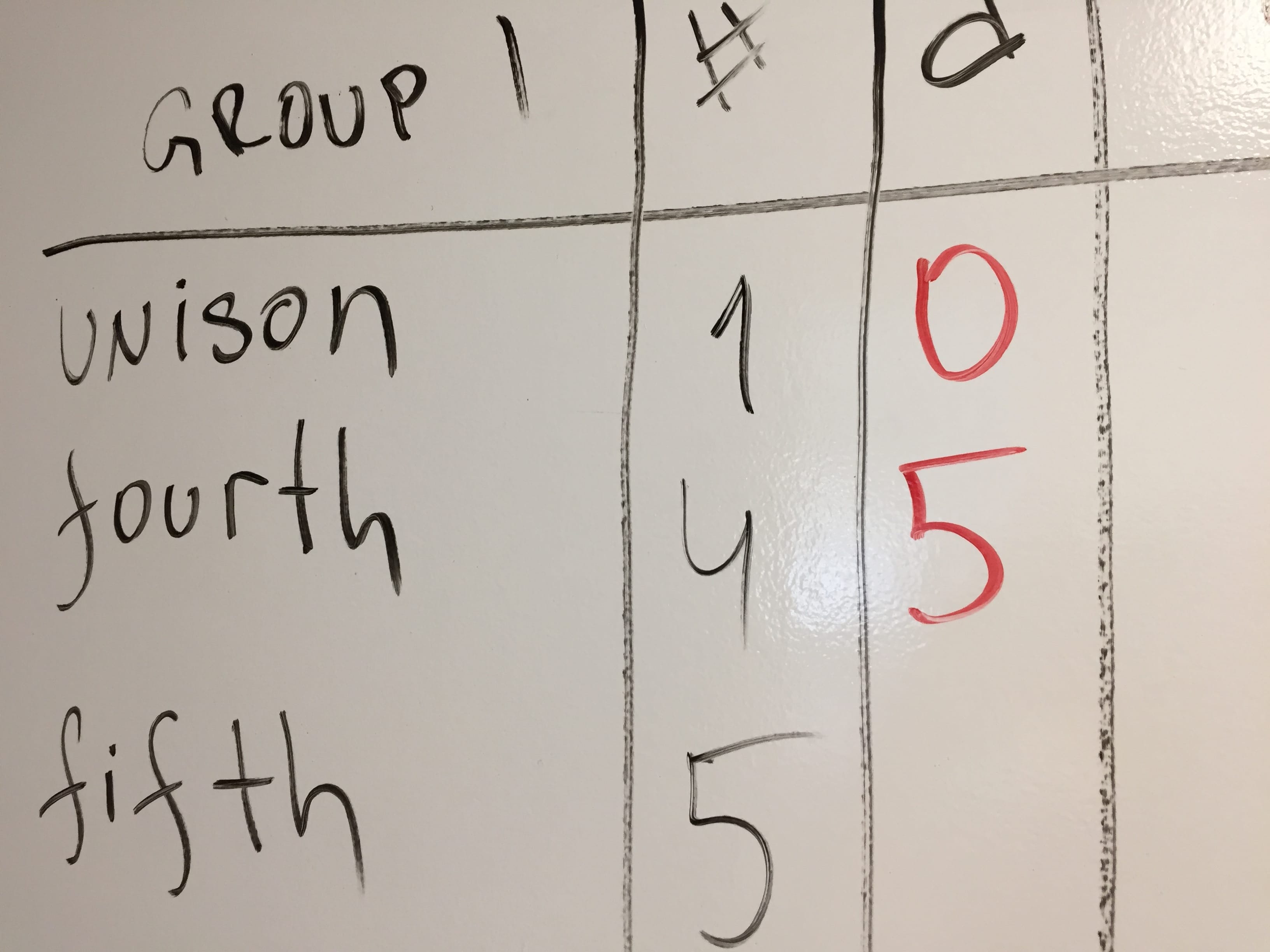
Let’s add some names to those numbers, starting with the oddballs: 1 is called unison and 8 is called an octave.

Unison means “the same sound”. So the first C and the first C are the same, the interval between them is a unison. Why 1 and not 0? Because when you count the number of lines and spaces you start with the first note. You put your finger on the first note and say “one”. As you move up, you increment. Don’t worry, you’ll see a lot more of this later.

Next, let’s fill in the rest of the interval names in the left column (group 1). They are pretty boring: fourth and fifth.

Now the right column (group 2). It’s all-too-boring too. Second, third, sixth, seventh.

Back to the discussion above, let’s learn to count lines and spaces.

Put your finger on the first note, say “one” and as you move up you increment for each line or space. So C is 1 and it’s on a line. You move up – it’s a space. You increment 1 – it’s a 2. The note there is D.

Next one up is E. It’s 3. F – 4. And so on.

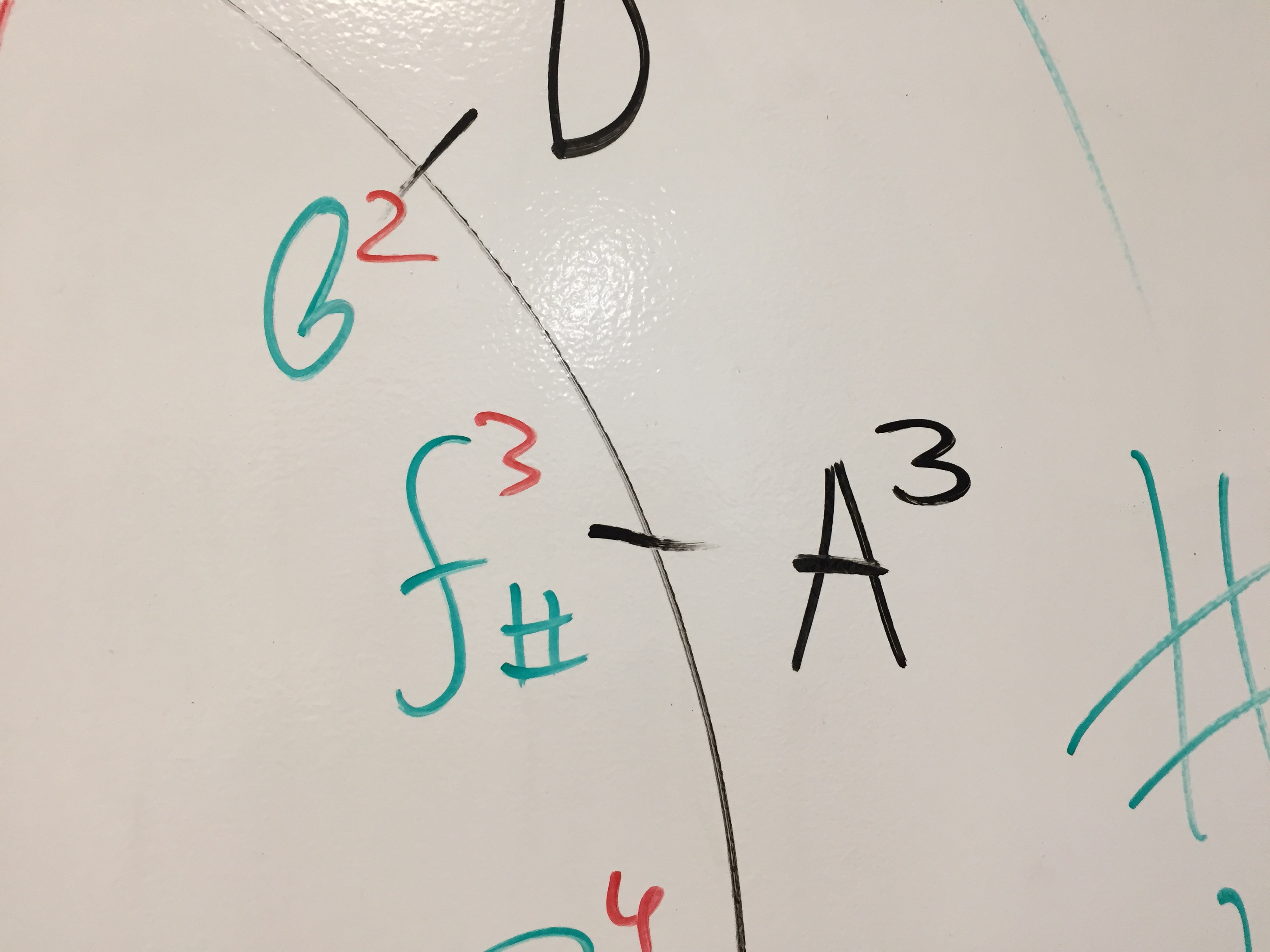
Now it’s time to populate the last column left – the one with distances in terms of semitones.

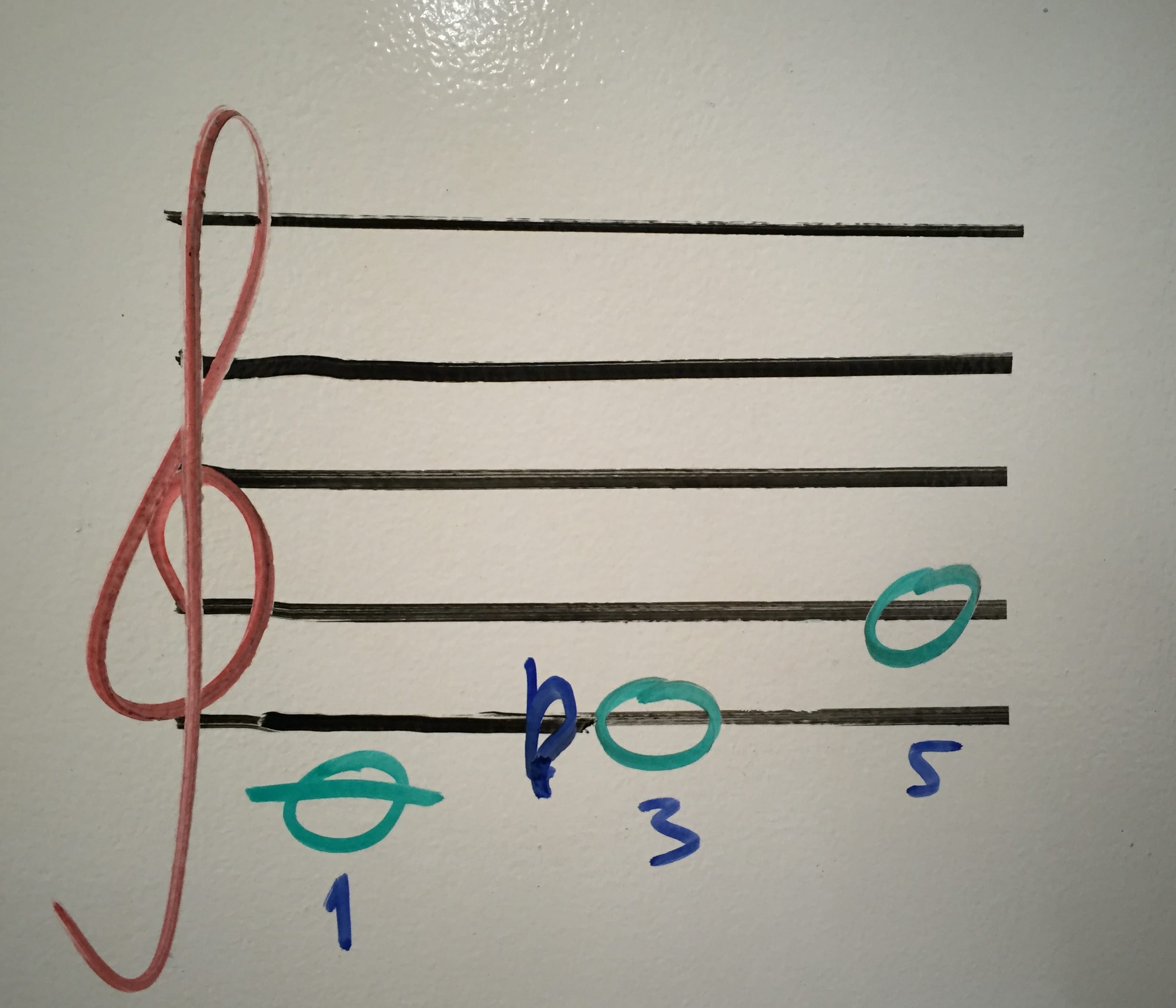
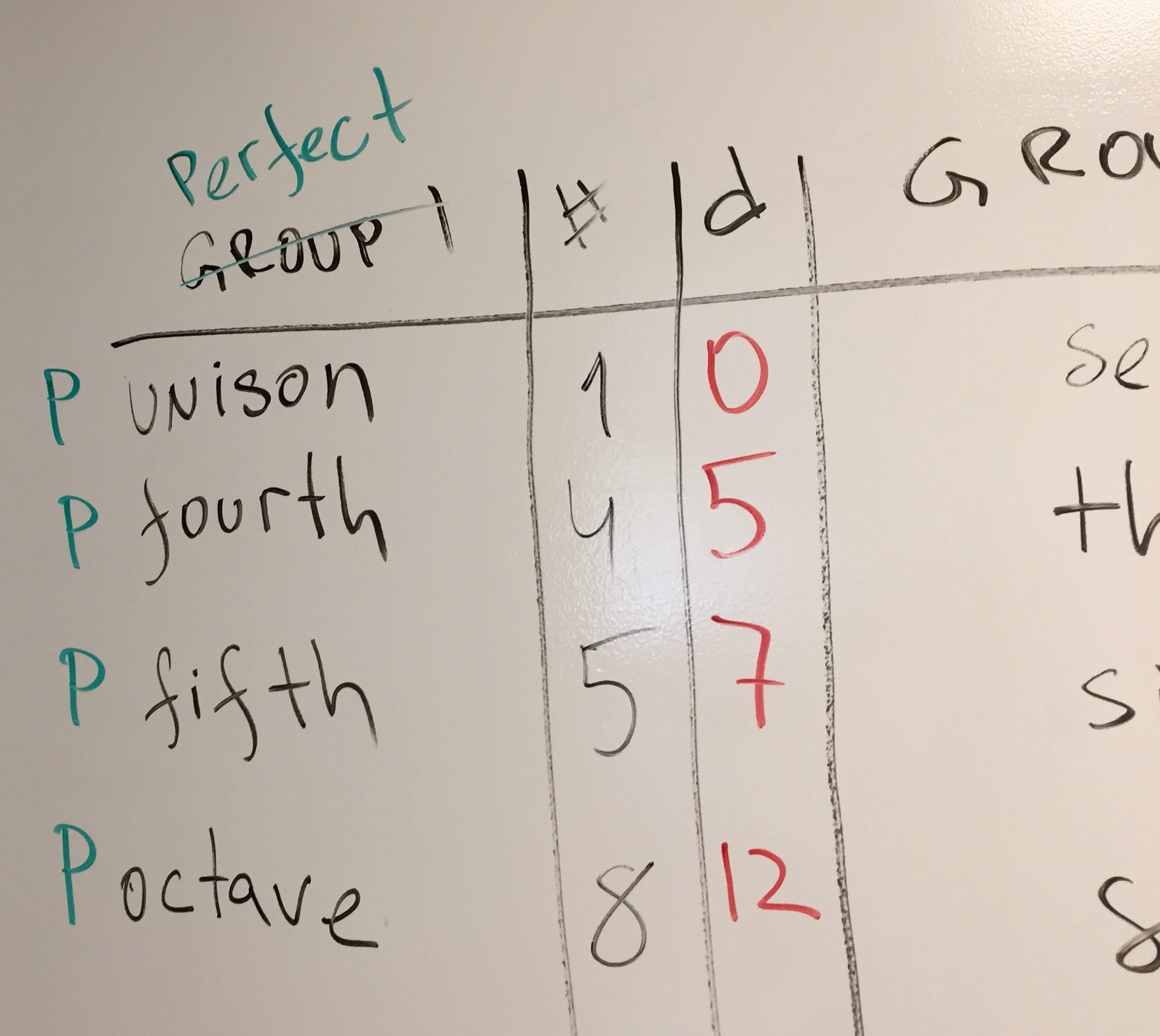
First is the unison. It’s the same C note. There are 0 semitones between the C and the same C. So put a 0.

Next comes the second interval called “second” (wow!).

The number of lines and spaces between C and D is 2, we already established this and put in the # column.

Now what about the distance in semitones? From C you go to C♯ which is one semitone, they you go to D which is another semitone. Two in total.

So since there are two semitones between C and D, you put 2 in the table.

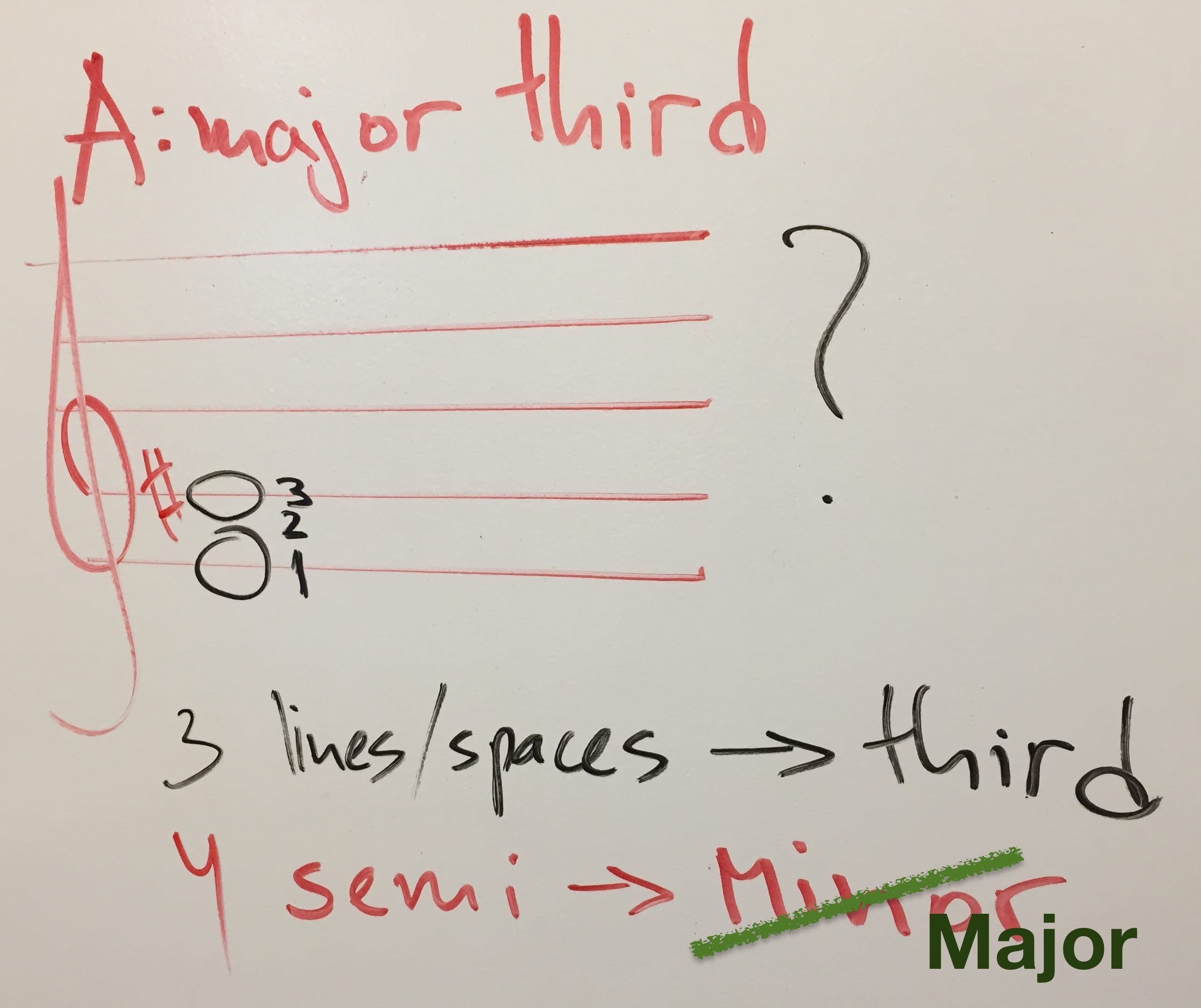
Next, the third interval. Between C and E. (BTW, we use C major to figure out the table because it’s the easiest, but these intervals then apply to any scale or starting point.)

There are 3 lines/spaces when you start from C and count C = 1, D = 2, E = 3.

And there are 4 semitones between C and E (C♯, D, D♯, E).

The semitone distance from C to E is 4 and that’s the number that goes in the table.

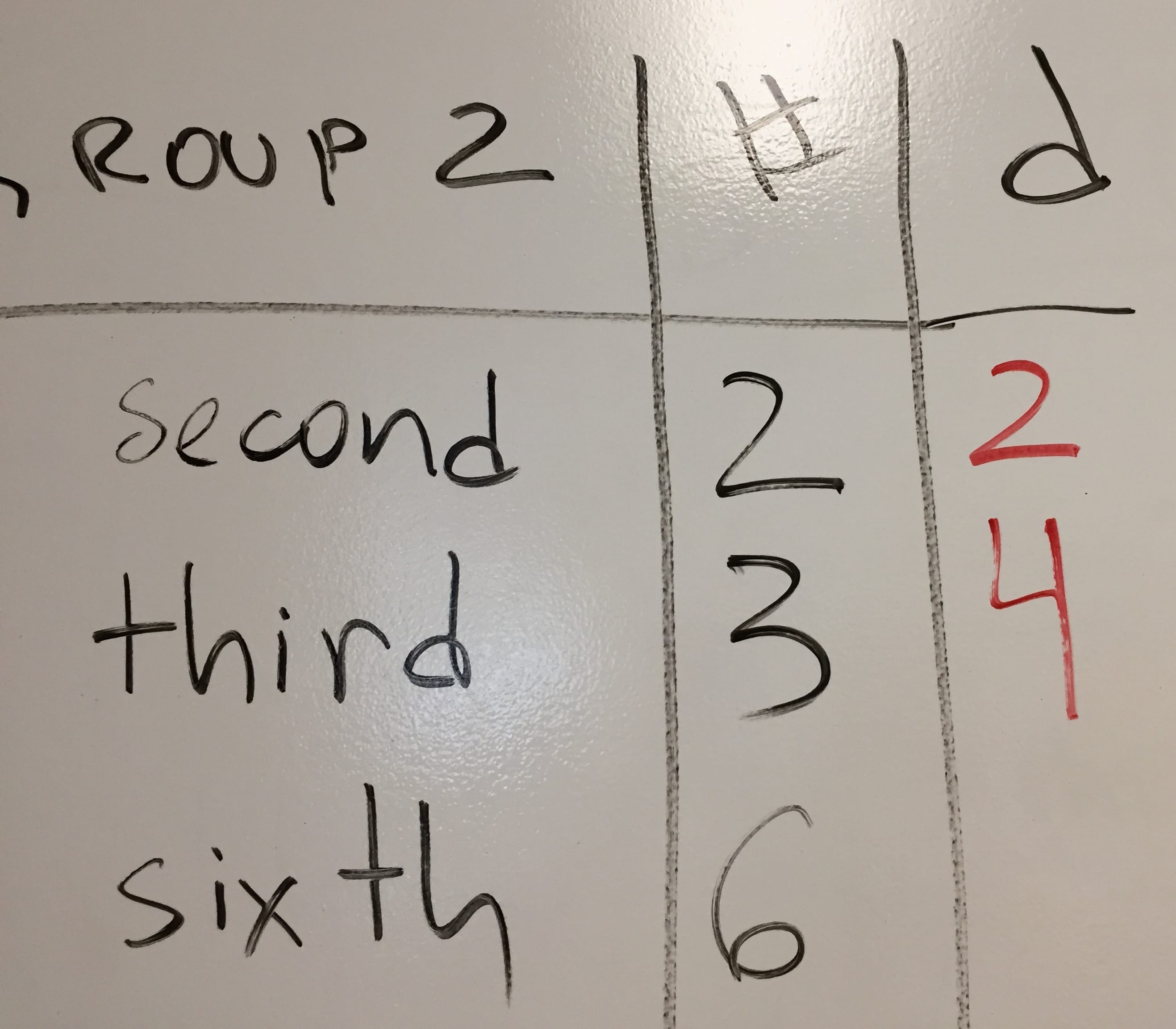
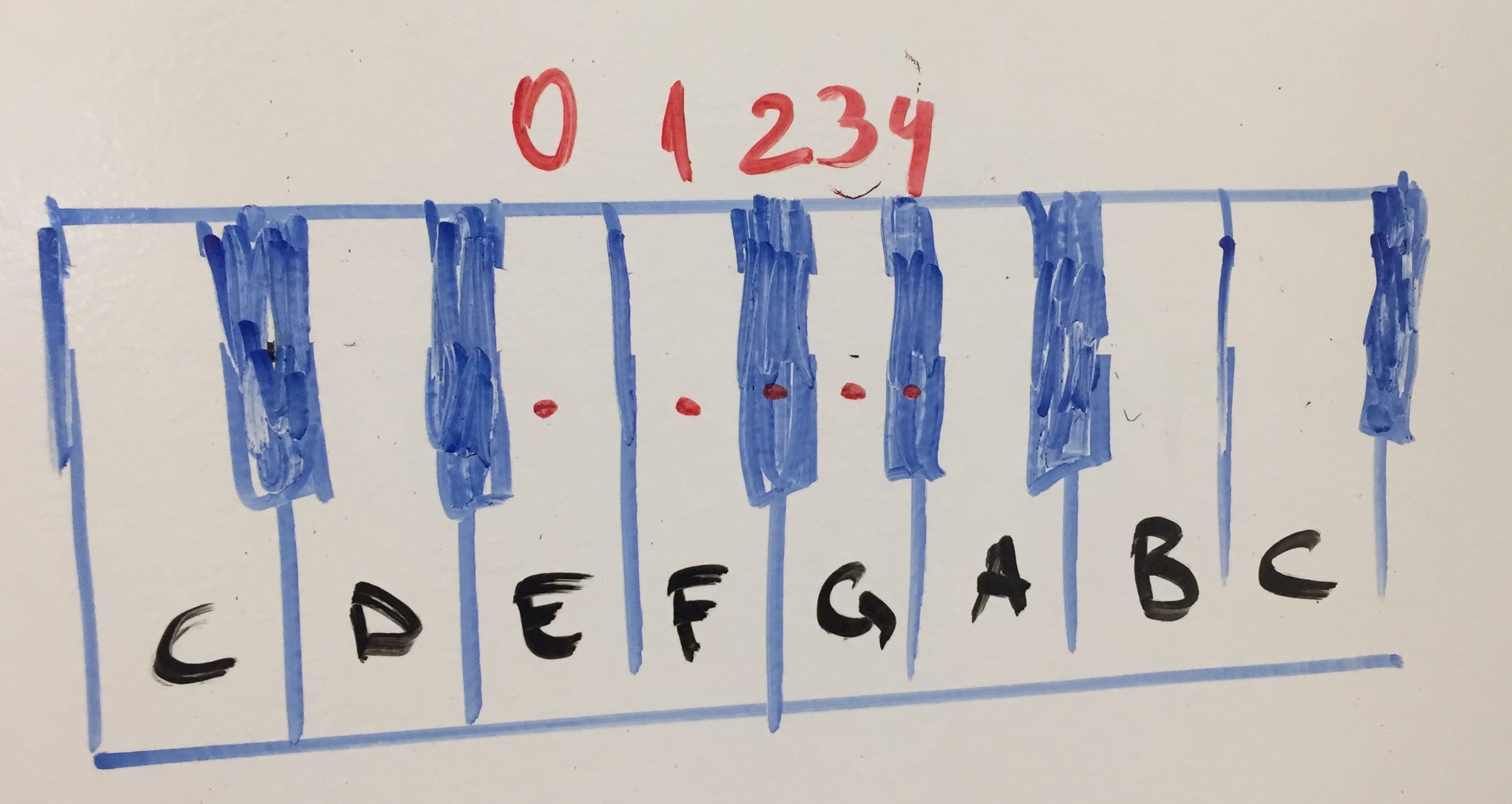
Next number if 5. Why? Look at the keyboard above and count the semitones between C and F.

Next number is 7. Why? There are C♯, D, D♯, E, F, F♯, G (7 in total) semitones between C and G.

The distance from C to A is 9 semitones as you can see keyboard picture above.

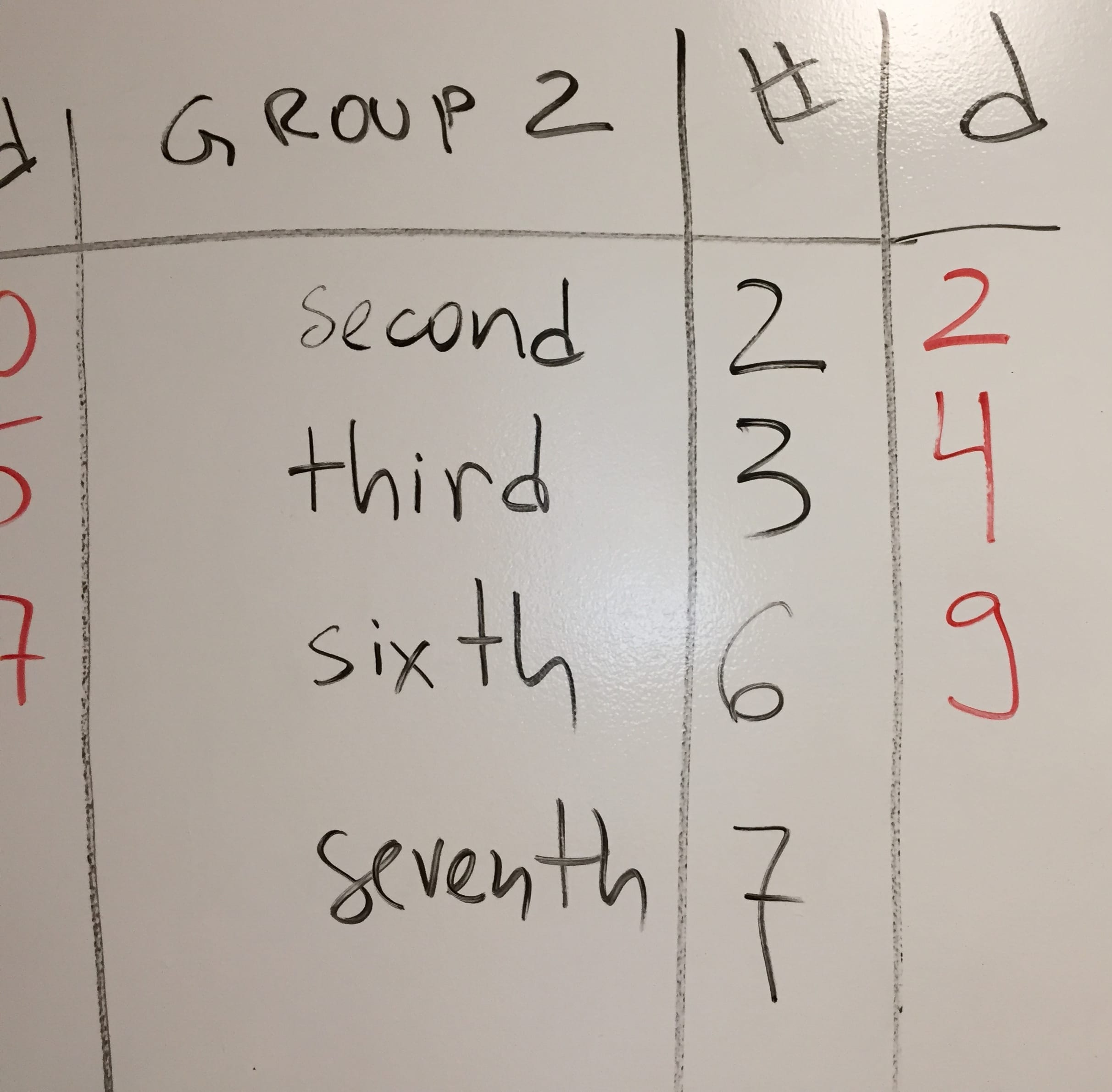
To wrap it up – C to B is 11 semitones and C to C is 12.

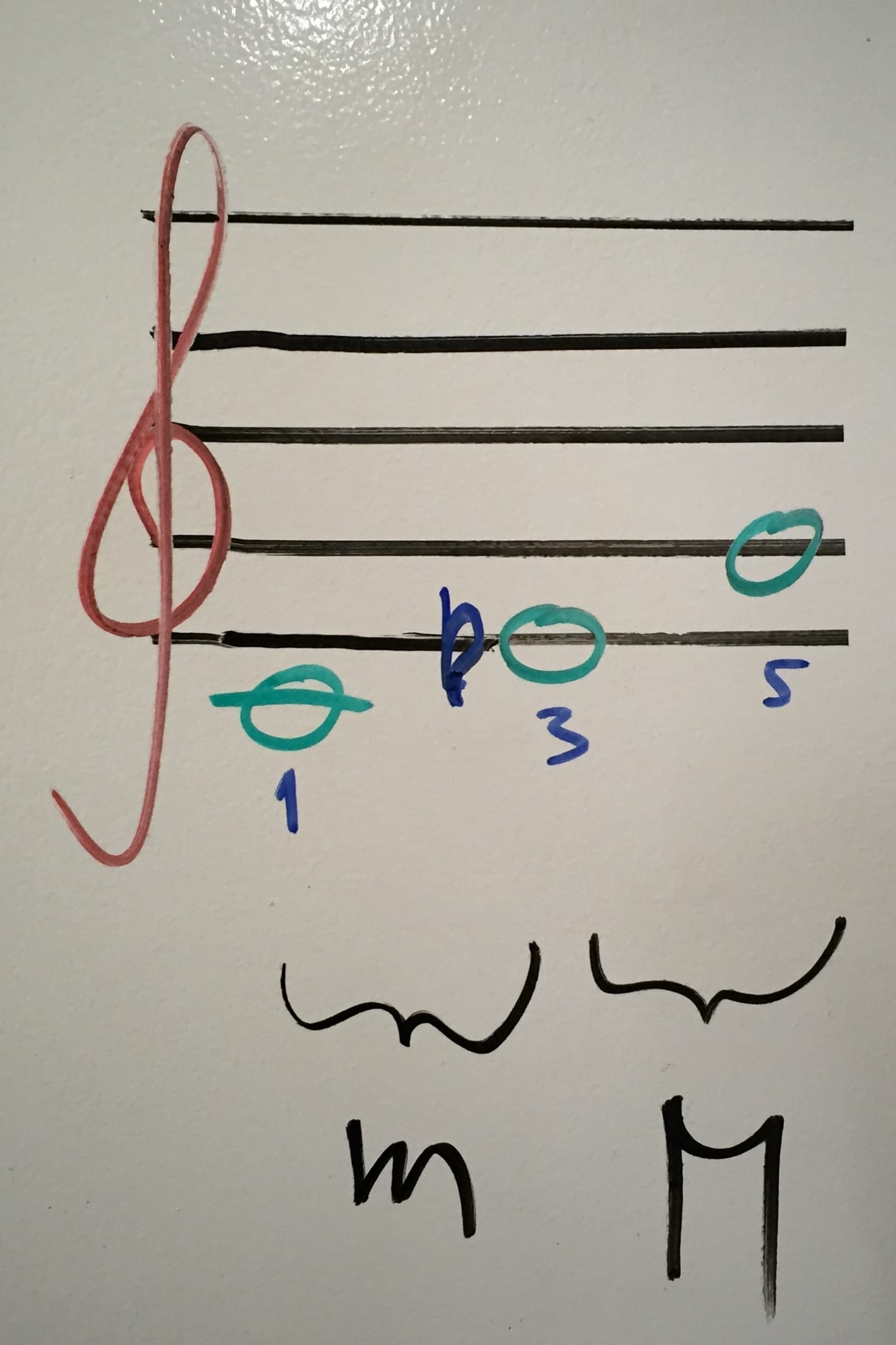
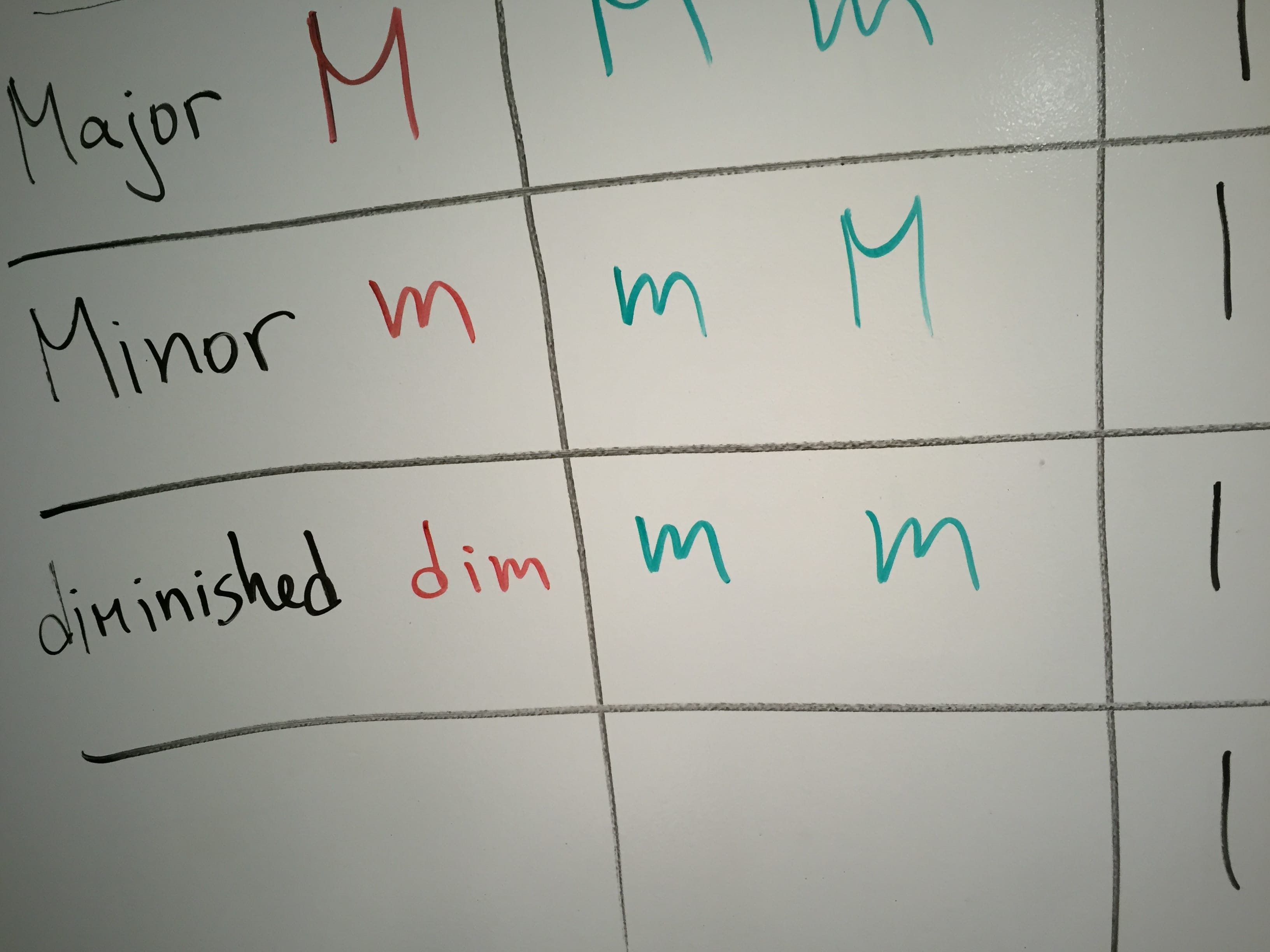
“Group 1” and “group 2” aren’t particularly cool names. We need something better. Let’s put a P in the left group.

P stands for “perfect”. Let’s call the whole group the perfect group.

The second group is called the Major group.

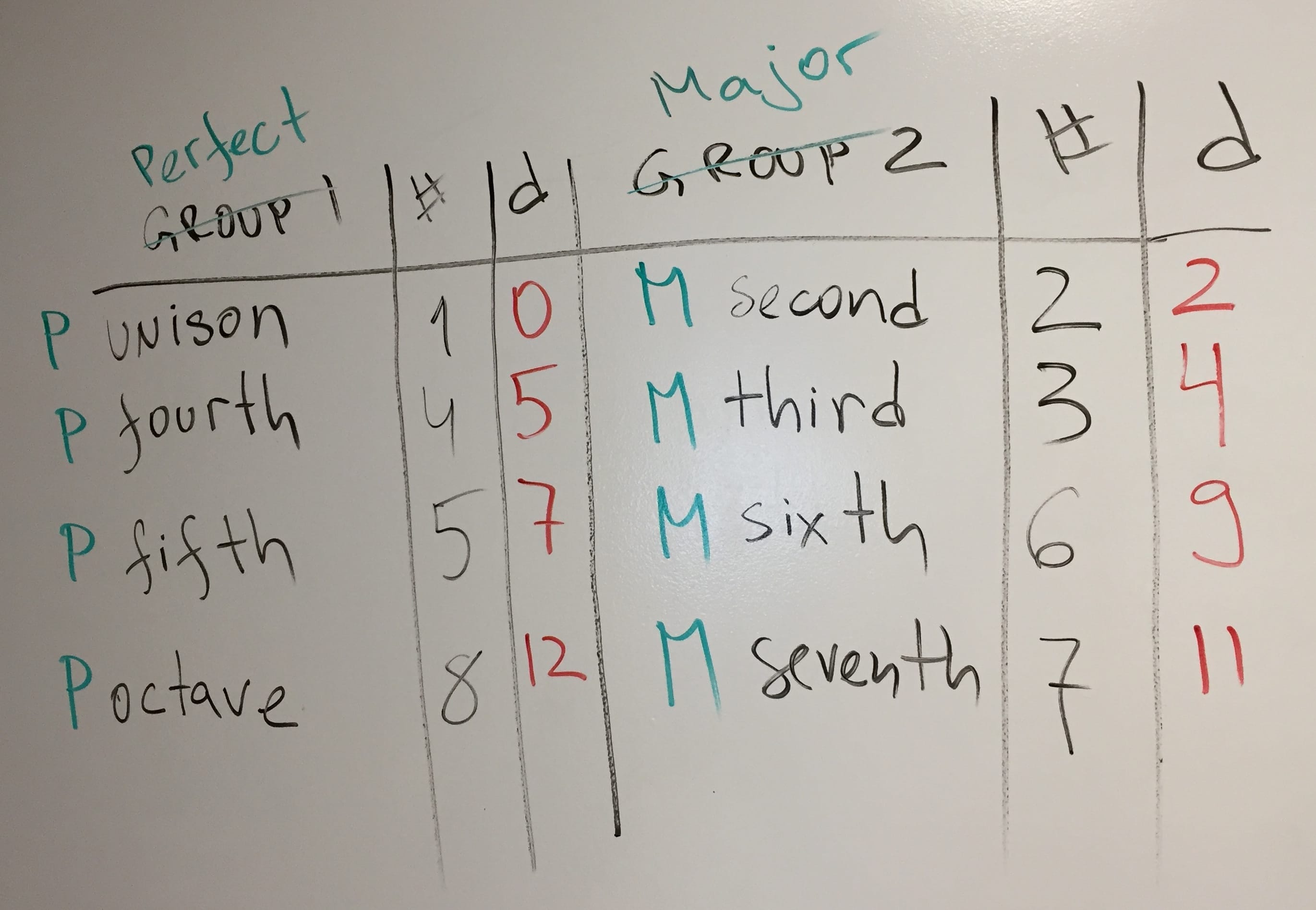
Now let’s stop here for now and see how to use what we have so fat in the next post.

Adios!

Aside: Why the name Perfect? Cool story.

Imagine a string. Pluck it. Now another string that’s exactly half as long the first. Pluck. The twice-as-short string makes a very similar sound only higher (an octave above). If you play the two together, they sound good even to the pre-historic human. Perfect actually. (Today we call it Perfect 8th.)

Now the unison is two strings of the same length vibrating. They sound the same and they sound good together. Boo-boo the Conqueror of the Cave as well as Bach both will agree they sound perfect together. (Only Bach would say the two sounds are in a perfect unison.)

If you shorten the second string to be 1/3 of the length of the first, the two still sound good together. A perfect 5th. Ask all heavy metal and punk guitar players – this interval is just perfect for most songs. When you play C and G together with a distorted guitar it sounds awesome. C and G#? Not so much.

If you shorten the string by a 1/4 and you play both they still sound good, albeit borderline. Pushing the limit of what your ear would call perfect. This is the perfect forth. Anything else is not so perfect. Therefore it needs a different name – let’s go with Major.

Guitar harmonics

Octave, fifth and fourth (the perfect) also happen to coincide (well, no coincidence really) with where the harmonics of a guitar string are easiest to produce. Harmonics is when you hold your finger but not press on the string above 12th, 7th and 5th fret. 12th fret (the octave) is the easiest and perfectiest, the 5th fret is borderline (the perfect fourth interval).

12th fret of the guitar is exactly the middle of the string, so no surprise.

Pentatonic? ‘nother cool story.

Pentatonic scale is 5 (penta) notes before you reach an octave. For the longest time in human history it was the only scale before our ears became sophisticated enough to find a few more notes musical. All Chinese music, all Scottish, all blues, all everything for a long while just used a pentatonic.

Example, common in blues guitar – A C D E G. 5. You know what’s cool? You make up this scale only using perfect 5th intervals.

C to G. G to D, D to A, A to E.

Think about this.

(If you’ve come to this page out of nowhere, please see the Circle of fifths explanations part 1, part 2, part 3)

Now that you know all there is to know about the Circle of fifths, it’s time for a little test… Identify the key signatures that you see in the pictures below. Every picture has a corresponding major and a minor key. Figure out one or better yet, both of them. To check your answers, click on an image. If I got any of them wrong, let me know I’m an idiot.

So far you know how to draw the circle of fifths using the old FCGDAEB (Fat Cats Go Down And Eat Breakfast).

You also know how to figure out which ♯s and ♭s you need to make up a major scale.

Goodie.

Now it’s time for minor scales.

Good news: you already know all there is to know. You use the same ol’ circle.

TL;DR: The only thing different is that the top of the circle (the North pole) is a (meaning a minor) instead of C (C major).

You picked up on Majors being Uppercase and minors being lowercase? Gooood.

K, let’s back up a bit.

Here’s the circle of fifths that you’ve learned to love and cherish (and draw by yourself too!):

Now instead of C at the top, you put an a. But on the inside of the circle:

It makes sense, doesn’t it?

C major scale is CDEFGABC – all the white keys on the piano. a minor is the same notes without any ♯s or ♭s. Still all the white keys on the piano. Only it starts from A not C. So the a minor scale is ABCDEFGA. But you probably knew that already.

So Major scales go outside the circle, minor scales go inside. They are minor. Fragile. In need of protection. Keep ’em inside.

Moving on.

If a is where C is, then a♯ should be where C♯ is and a♭ is with C♭.

Now go back up and finish “..And Eat Breakfast”.

Since you run out of the fat cat sentence, you start over with f. Only that all are sharp now (because you just finished with non-sharp, nor flat ones). In other words you write f♯, c♯, g♯, d♯.

a♯/a♭ is already there, you continue with Eat Breakfast (e♭, b♭). They are flat because they are on the flat side of the circle.

Then you start over with non-sharp/non-flats – f, c, g, d.

This is actually the full circle. It shows both Major and minor scales.

Almost. The problem is I forgot to take a photo of the numbers next to each minor letter. But you’re a smart cookie, you can figure those out. They are exactly the same as the Majors. a has 0 flats/0 sharps. Just like C. Then you move to the right and put 1 next to e. 1 sharp in e minor. (Just like 1 sharp in G major). And so on…

Now it’s test time. What is the signature of a minor?

Find it in the circle. Look at the number. It’s 0. No flats, not sharps.

There you go:

Something more interesting? f♯ minor.

Find it in the circle. It’s on the sharp side. And has the number 3.

So f♯ minor scale should have 3 sharps? Which ones? Back to the Fat Cat… (FCGDAEB).

The first three are F, C, G.

Therefore the signature looks like:

Or if you want to spell out the whole scale…

Thanks for reading!

Now you know how to draw the circle of fifths. And figure out ♯s and ♭s in all the major and minor scales. Hooray!

(Psst, practice now!)

Want some other things to practice?

Practice saying ABCDEFG aloud. You probably have no problem with this. It’s the alphabet.

Now start with B = BCDEFGA. Now start with C. And so on.

Now the same but backwards. Say quickly GFEDCBA. DCBA. DBA. DCBAGFED. And random stuff like this.

Now FCGDAEB. (Fat cat…)

Now BEADGCF.